Question about the relationship between the variance of residuals and variances of the data points

Question

I was reading about regression. I understand one of the how the variance of each $x_i$ needs to be normal distributed, but why doesn't that related to the $s_b$? It seems like it should. I was reading the AP College Board paper about Inference.

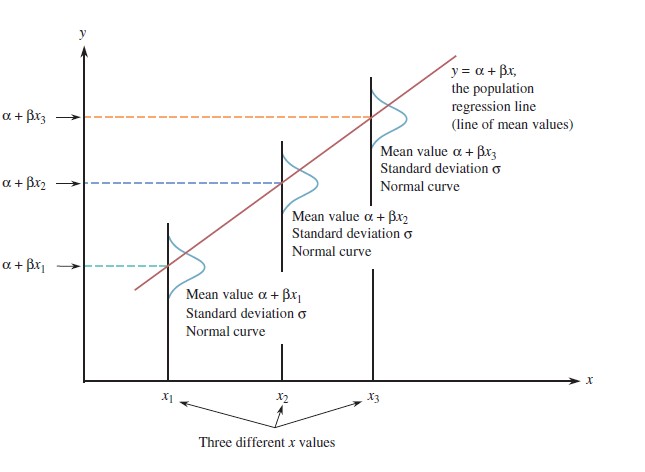
Two more assumptions are that the errors are normally distributed and that they have the same standard deviation for all x values. Although it is not at all obvious, this is not the same as saying that the residuals are normally distributed and all have the same standard deviation. The difference is that the “errors” are the deviations between the response variables and the “actual” underlying (invisible) linear phenomenon, whereas the “residuals” are the deviations between the response variables and the least-squares regression line, which is only an estimate of the “actual” line.

I understand (I think) that just because the error of the regression is normal doesn't mean that the variance of $x_i$ is normal because the sample could just randomly follow normality. (don't know if I said that statistically correct), but if all the $x_i$ are normal and the variance are equal, then why doesn't that variance equal the variance that one would get for the $\beta$

I have gotten some great answers here that are very clear and I am hoping that I could get another answer to help clear up my confusion.

The kernel of my question is What exactly is the relationship between the variance of the residuals and the $x_i$ and is there any connection to the error, variance of $\beta$

Answer 1

What the text you quote is saying is that in a regression model like

$$y_i=\alpha +\beta x_i +\varepsilon_i$$

whereas the true errors are given by

$$\varepsilon_i=y_i-\alpha-\beta x_i$$

the residuals are estimates of the errors given by

$$\hat{\varepsilon}_i=y_i-\hat{y}_i=y_i-\hat{\alpha}-\hat{\beta} x_i$$

If the errors are correctly assumed to be independently distributed according to an Normal distribution with zero mean and variance $\sigma^2$, that assumption doesn't hold for the residuals. Look up Studentization of residuals for more info.

What you seem to be asking is about the variance of the independent variable $X$. The regression model is fitted conditional on whatever $x_i$ you happen to set, or to observe, so the distribution of $X$, even if it is a random variable, doesn't affect the validity of the regression model. The particular arrangement of the predictors $x_i$ will affect the variance of the residuals, but not the errors, through the hat matrix - residuals at extreme predictor values will tend to have smaller variance. It will also effect the variance of the coefficient estimates $\alpha$ & $\beta$ through the information matrix - a bigger spread of predictor values determines the coefficients more accurately.