Consider a complex normal variable $Z \sim \mathcal{CN}(\mu,2\sigma^2)$ with real component $X \sim \mathcal{N}(\mu,\sigma^2)$ and imaginary component $Y \sim \mathcal{N}(0,\sigma^2)$. We can write joint probability function of real and imaginary components as bivariate normal distribution $$ f(x,y|\mu,\sigma) = \frac{1}{2\pi\sigma^2}\exp{\left(-\frac{1}{2}\left(\frac{x-\mu}{\sigma}\right)^2 -\frac{1}{2}\left(\frac{y}{\sigma}\right)^2\right)}. $$
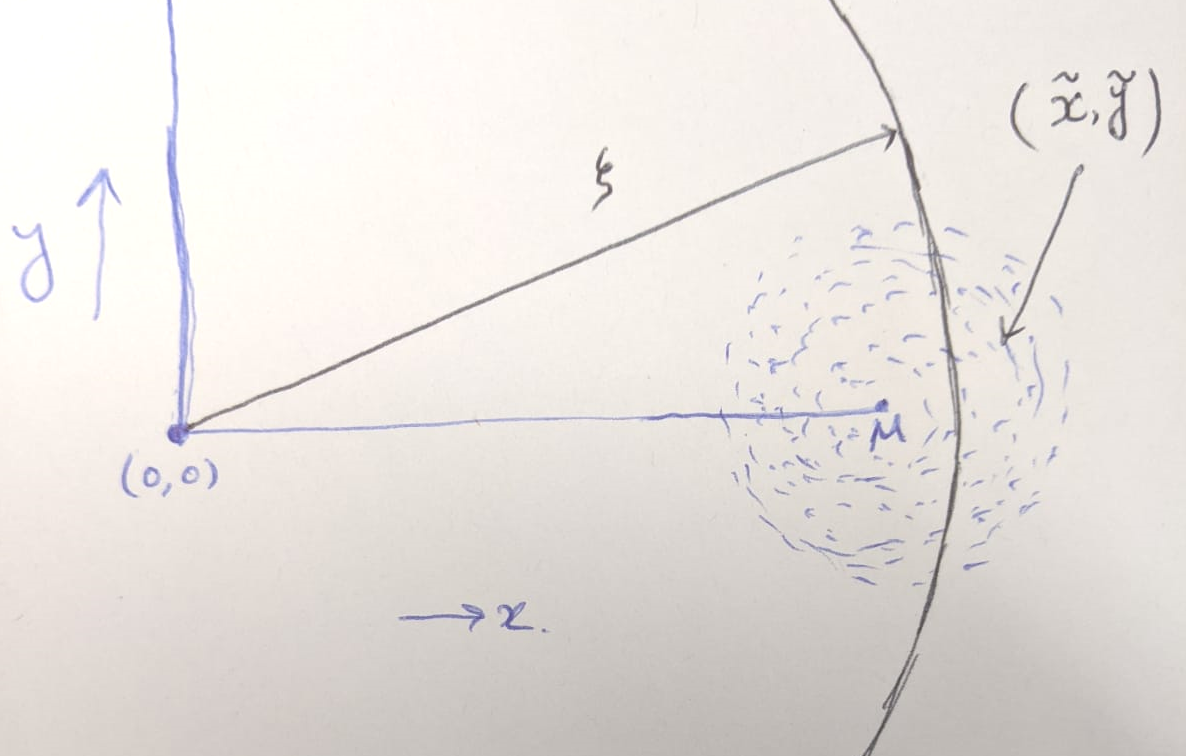
Consider $\tilde{x}$ and $\tilde{y}$ are real and imaginary components where $x^2 + y^2 > \zeta^2$, so we can write this as conditional probability function $$ f(\tilde{x},\tilde{y}) = f(x,y|x^2 + y^2 > \zeta^2).$$
Here, $\zeta$ is a real constant threshold value i.e. $\zeta \ge 0$. I am interested in variance of $\tilde{x}$ and $\tilde{y}.$ What I have understood, I need to compute first $f(\tilde{x},\tilde{y})$ and after margilization I can get $f(\tilde{x})$ and $f(\tilde{y})$, from there variance can be computed. Can you please help me to find $f(\tilde{x})$ and $f(\tilde{y})$. Any approximation will also work. To put things in perspective, I have attached figure.