0
$\begingroup$

enter image description here

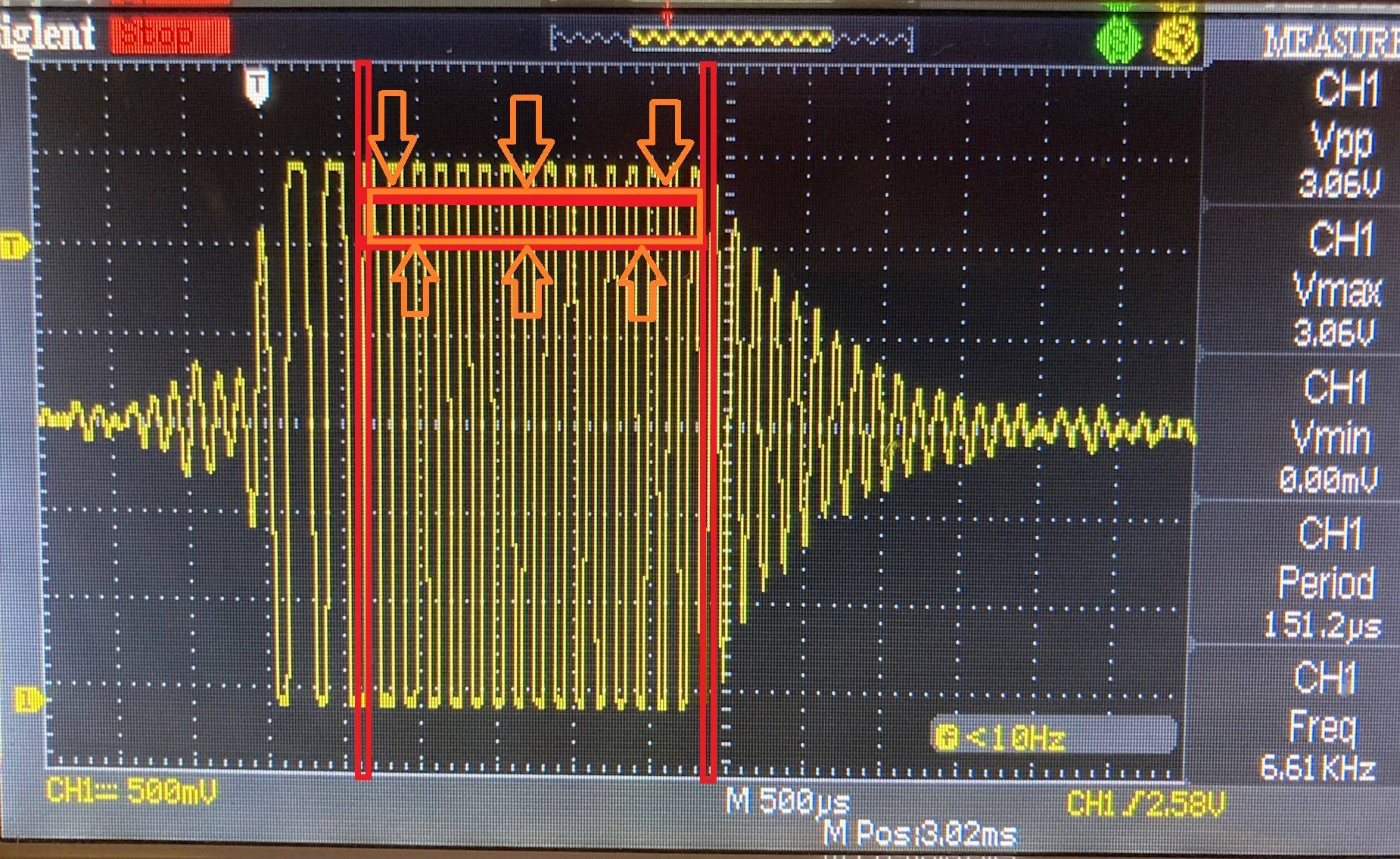
EDIT 3: added below picture of raw readings of oscope from doppler data showing "periods" of sine waves before processed sine signal into square pulses. enter image description here

EDIT 1: These numbers on the left are period time values in micro seconds (us) in the "time domain" and they need to be converted to the "frequency domain" Sampling rate was 76800 hz and the total length of sampling was 23682500us or 0.00236825 seconds. The actual frequency I am seeking is 6660.984 Hz (or close to it) which is 150.1279us period.

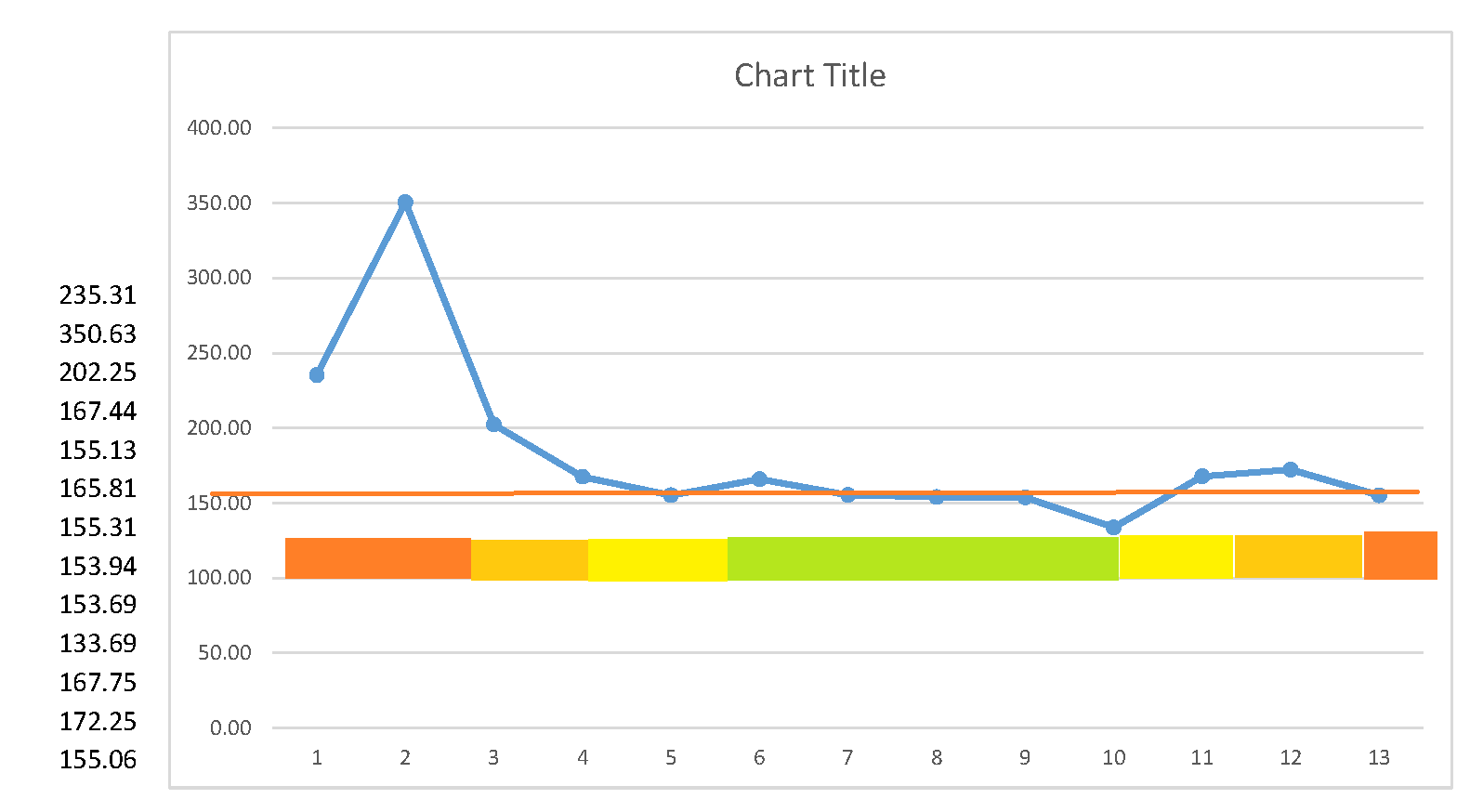
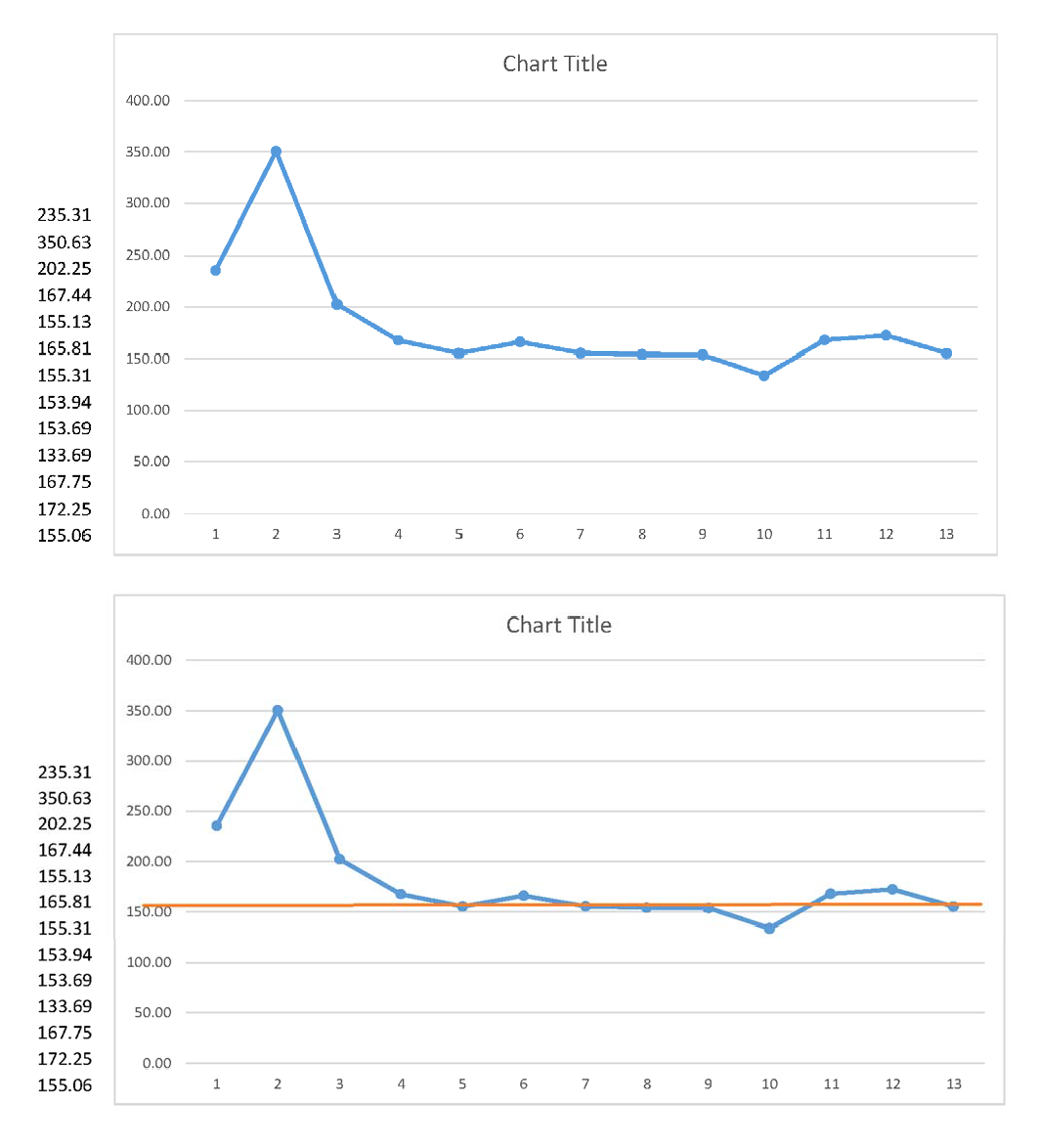
This is the result of a doppler reading of time values that will be converted to frequencies. The numbers are listed on the left side of the graph.

Would like to find the dominant value. I think FFT/FHT may be the solution, but do not think there are enough data points.

Want to arrive to as near as possible to the solution: i.e. the Actual Number the red line in the graph.

What numbers to expect in the string?:

The number of data points will be between 5 and 20. (in the vast majority of cases between 10 and 20 data points)

The will be long integers (for example: 1359375) that I will convert into fractional with up to 5 decimal spaces (like 135.9375).

The data line string will always start with values (2 to 6 data points) that are about 10-30% higher than the Actual Number and will progressively decrease and settle into a horizontal level line.

The Actual Number’s value will be very close to the values of the data points that are located from the 50% (middle) to 80% part (near the end).

There will be at least 2 to 6 data points in that area that will be within 5-10% from each other and also very close to the Actual Number.

The data points will never exactly match each other because they contain up to 5 more fractional digits. So MEAN averaging would not work unless able to “round” them up first.

I believe weighed moving average would work best. But that’s because I’m not familiar with other statistics options.

EDIT 2: added color chart of areas where the numbers are most likely to be closest to the actual value I am seeking. enter image description here

Improve this question
$\endgroup$
4
  • $\begingroup$ Suppose you get 7 values near 200 and 6 values near 330. Do you want to conclude that there is a dominant value near 200 or that there is a dominant value near the mean of 260? Both are plausible conclusions, depending on the underlying model or the expected distributions. $\endgroup$
    – user225256
    Commented May 31, 2021 at 18:03
  • $\begingroup$ @Matt F. Which ever values (200 or 330) are located inside the green zone should get a higher weight (linear, exponential?) vs the ones inside the yellow zone. But values inside the yellow zone (200 or 330) should get (linear, exponential?) higher values vs the ones in the orange zone, and so on with the red zone, etc. Yes, any values inside the "high-probability" zones, regardless if 200 or 330 should be considered. If it's near even like 6 (200) vs 7 (330) then maybe proportionally "near" mean? $\endgroup$
    – TommyS
    Commented May 31, 2021 at 18:27
  • $\begingroup$ That’s not clear enough to decide on a statistical test or procedure. What are the values measuring? And what is the model or set up that makes you expect a dominant value? $\endgroup$
    – user225256
    Commented May 31, 2021 at 18:35
  • $\begingroup$ EDIT: These numbers on the left are "period" time values in micro seconds (us) in the "time domain" and they need to be converted to the "frequency domain" Sampling rate was 76800 hz and the total length of sampling was 23682500us or 0.00236825 seconds. The actual frequency I am seeking is 6660.984 Hz (or close to it) which 150.12us period. $\endgroup$
    – TommyS
    Commented May 31, 2021 at 18:47

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.