13
$\begingroup$

I'm going through the Dragonnet paper (slides available here), and the authors use Pearl's do notation to make this claim:

enter image description here

How can I interpret the do notation? Is the author claiming that the average treatment effect is the difference between the expectation of the outcome given that the entire population is treated and the same expectation given that the entire population is not treated?

If so, why the conditioning on confounding effects would remove this need?

Improve this question
$\endgroup$
4
  • $\begingroup$ By the way, the authors of your slides use the unfortunate term "Covariates" for the confounding variables. A confounding variable IS EMPHATICALLY NOT a covariate. There are lots of covariates that are not confounders, and no doubt you can come up with confounders that don't co-vary. The back-door path criterion is THE DEFINITION for a confounder, period. $\endgroup$
    – Adrian Keister
    Commented Jun 8, 2021 at 18:04
  • $\begingroup$ What I do not understand about the do notation is what "forcing X to have value 1" corresponds to in the real world. Is it the difference between an individual choosing to smoke versus being forced to smoke? Or is it the difference between an entire population choosing to smoke versus being forced to smoke? Or both? But then since we only have individuals in an observational study who choose to smoke how could we ever estimate the effect of being forced to smoke without assuming they are the same. $\endgroup$
    – stataphobia
    Commented Sep 24, 2021 at 23:25
  • $\begingroup$ It's the difference between the entire population smoking or not smoking. And you have to specify a structural causal model or at least a DAG with variables on which you defined the causal effects using $do$. If you think there is a variable "being forced to smoke" and a separate variable "smoking", then you need to include them in the model. $\endgroup$
    – Julian Schuessler
    Commented Sep 29, 2021 at 7:51
  • $\begingroup$ Thanks Julian. That is helpful. So then does this mean we are not making a consistency assumption which would say the effect of smoking on Y is the same regardless of how the smoking variable is set? Or is it that the consistency assumption only holds at the subject level, and the DAG is giving you relationships between variables at the population level? $\endgroup$
    – stataphobia
    Commented Sep 29, 2021 at 21:27

1 Answer 1

Reset to default
17
$\begingroup$

The proper interpretation of the do notation is that the expression $\operatorname{do}(X=1)$ means you are forcing $X$ to have the value $1.$ You are intervening to make that happen. In the Directed Acyclic Graph (DAG) context, the notation $\operatorname{do}(X=1)$ means you do three things:

  1. Delete all arrows going into $X.$
  2. Replace the node $X$ with the value $1.$
  3. Update the Structural Causal Model accordingly. This assumes you have a Structural Causal Model.

In an experimental context, it is commonplace to force variables (or factors) to be certain values in order to eliminate unwanted variation. One of the many benefits of Pearl introducing the $\operatorname{do}$ notation is that we can now codify experimental assumptions and procedures in a framework that's easy to analyze.

You wrote:

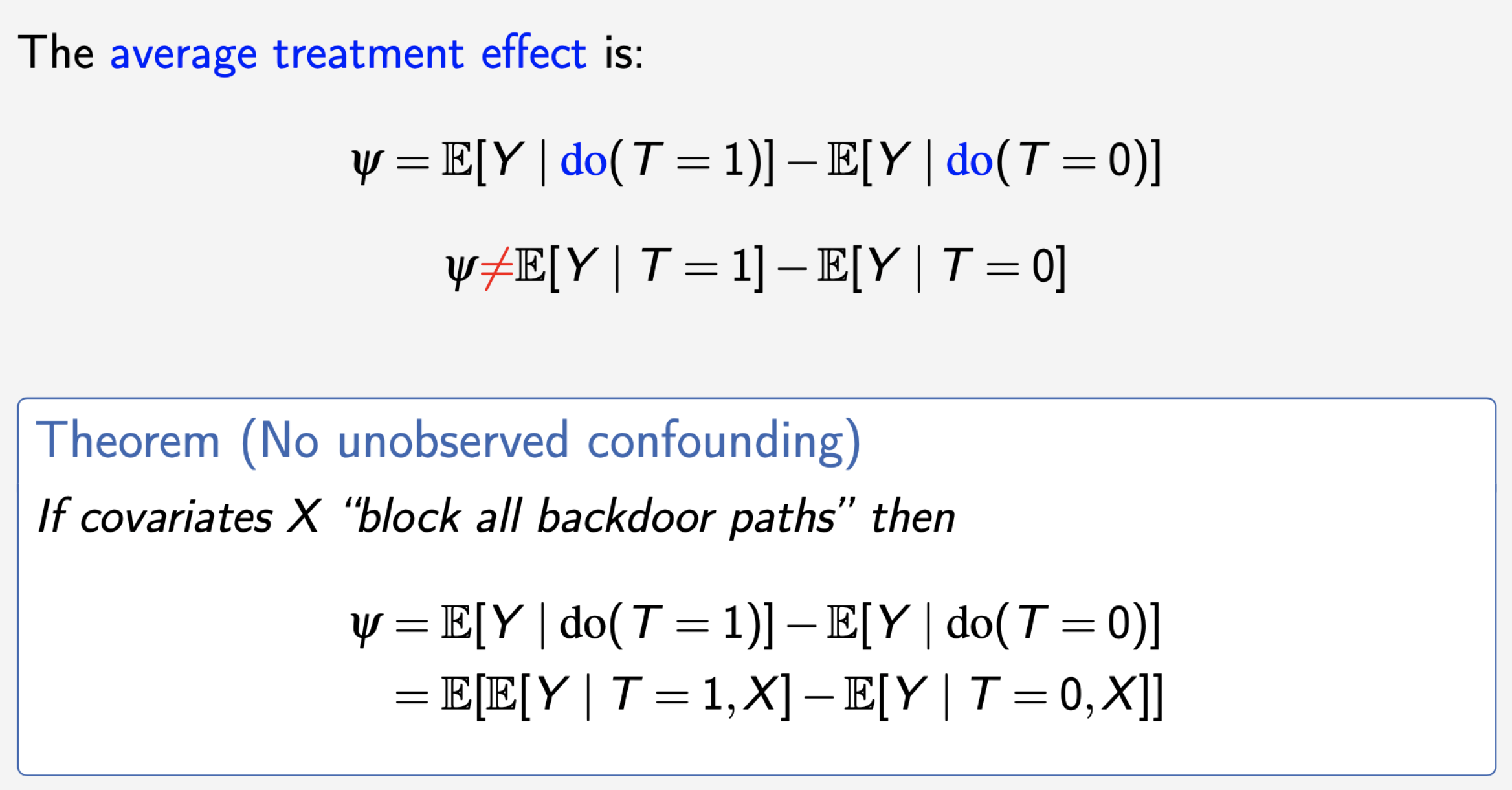
Is the author claiming that the average treatment effect is the difference between the expectation of the outcome given that the entire population is treated and the same expectation given that the entire population is not treated?

The answer is "yes", if you interpret your words "is treated" as $\operatorname{do}(X=1)$ and "not treated" as $\operatorname{do}(X=0).$ The main goal of the do-calculus, the back-door criterion, the front-door criterion, instrumental variables, etc., is to reduce causal expressions containing the do operator to probabilistic expressions that do NOT contain the do operator. That way, you can evaluate the result using your data.

The problem is that, in general, $P(Y|\operatorname{do}(X=x))\not=P(Y|X=x).$ So you have these procedures that Pearl and others have come up with for removing the do operator from your expression.

You wrote

If so, why the conditioning on confounding effects would remove this need?

Read The Book of Why or Causal Inference in Statistics: A Primer to find out why this works. Indeed, the fact that we really know what a confounding variable is (a variable that sets up a back-door path from the cause to the effect) is one of the supremely valuable things the New Causal Revolution has given us.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.