1
$\begingroup$

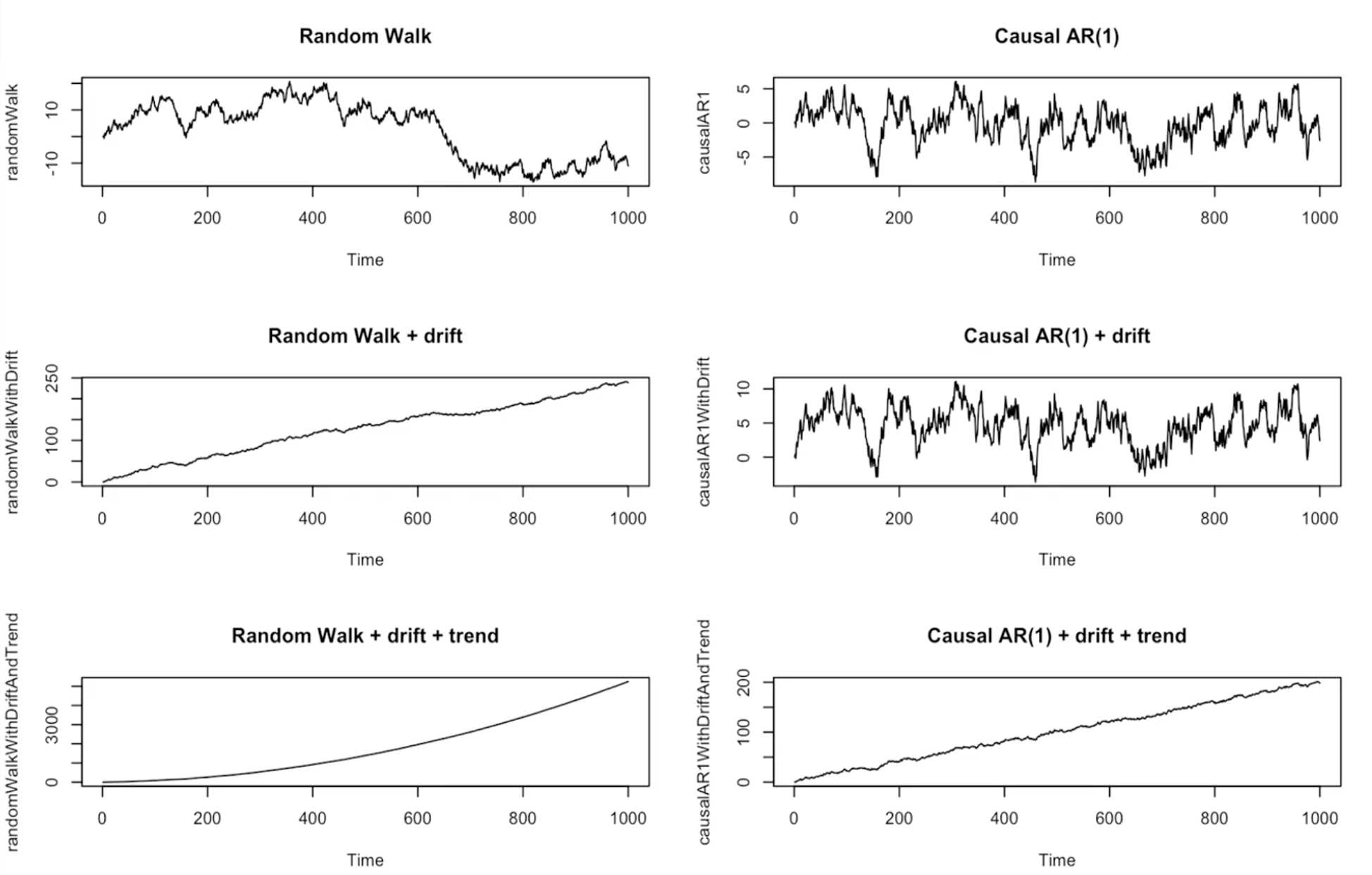
I'm a bit confused about the definition of weakly stationarity

from looking at the definition of weakly stationarity, it requires: E(xt) = E(xt−j ) = µ ∀ j var(xt) = var(xt−j ) = σ^2 ∀ j cov(xt, xt−j ) = γj ∀ t

But from looking at the graph of causal AR(1) + drift + trend, I don't think the E(xt) is constant (mean is increasing as t increases), also the variance is not constant from the graph, so why is causal AR(1) + drift + trend weakly stationary? enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ Hi: if you add a trend to a weakly stationary series ( in other words, a $b \times t $ term ), then the process could be termed trend stationary which means that, if you de-trended, you'd get a weakly stationary process. But, I don't see how you can tell that the variance is not constant ? Also, it would be better if you wrote out the whole equation so that it was clear what you meant by drift ? Are you just adding a constant to the process ? $\endgroup$
    – mlofton
    Commented Jul 5, 2021 at 5:49

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.