Meaning of multiple exposures in a DAG

Question

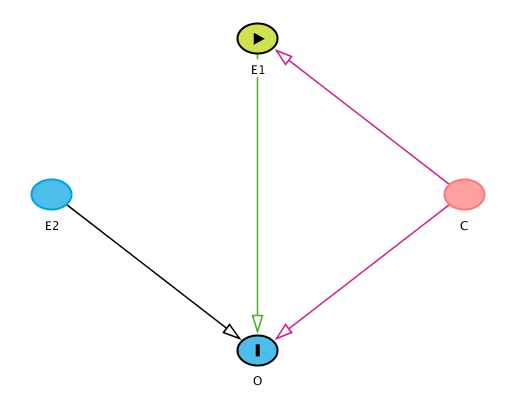
I am working with DAGs as a way to do some causal modeling. I am using dagitty - both the website and the R package. I feel like I have a good grasp of most things related to confounding, adjustment sets, and so on. However, something has me confused. Let me demonstrate by example.

Assume I have the DAG

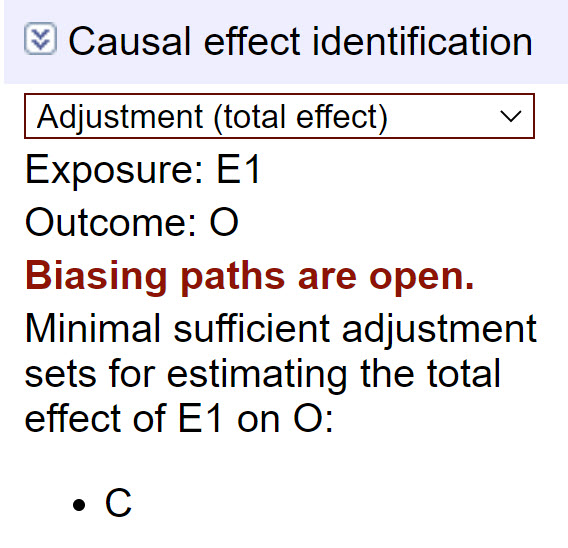
I understand (and dagitty confirms) that to extract a causal value for E1 I need to adjust for C. Dagitty reports "

So, for example, if everything was linear, I would build a model O ~ E1 + C, and I would intepret the coefficient of E1 as causal. I understand that I cannot interpret C as causal - this would violate the "Table 2 Fallacy".

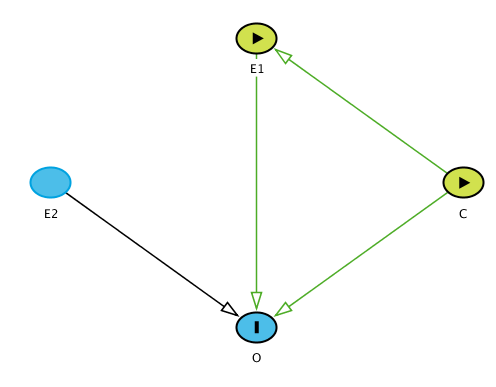
Now for the part that confuses me. Dagitty allows a user to pick multiple exposures. So, for example, I can choose both E1 and C as "exposures".

Dagitty now tells me that "No adjustment is necessary to estimate the total effect of E1,C on O." This would seem to suggest that the same model: O ~ E1 + C can be used, but now ?both? E1 and C have casual meaning. Obviously, this is wrong.

My question is - what is the meaning of "the total effect of E1,C"? Since C causes E1 (by my diagram) I can't set them independently. More generally, what is the meaning of any multiple exposure selection. In other words, let's say I had the DAG E1 -> O <- E2. What would be the meaning of setting both E1 and E2 as exposures?

Thanks in advance for any explanations. Every time I think I have my head wrapped around causal inference, some new "wrinkle" pops up.

Answer 1

The problem is the same estimation procedure you used in the first case, cannot be used in the second.

DAG 1:

In the first case, you rely on a very special case where the average causal effect (ACE) can be estimated. Specifically, if (1) the outcome is continuous, (2) the true model specification has not interaction terms, and (3) a single exposure; then the coefficient for $E1$ can be interpreted as the ACE for $E1$ on $O$. As you say, trying to interpret the coefficient for $C$ leads to the Table 2 Fallacy.

A more general approach (i.e., it works when the outcome is not continuous or when there are interaction terms) is g-computation. Briefly, we would estimate the outcome model as suggested. Then we would manually set $E1 = 1$ in a copy of the data set and use the fitted model to predict $O$ for everyone with $E1$. This process would then be repeated for $E1=0$. Then we would take the mean of those predictions under each set $E1$ and then subtract. This gives us the ACE while allowing for more complex models. Furthermore, it prevents the Table 2 Fallacy by only providing the ACE as the output.

DAG 2:

Now in the second example, you try to use the linear regression trick but it won't work in this case. There are now two exposures that we are setting. Specifically, we may want to estimate the ACE had everyone been given $E1=1,C=1$ versus had everyone been given $E1=0,C=0$. The linear regression coefficients cannot give you these comparisons (since they condition on the other). Trying to interpret these issues again would end up with the Table 2 Fallacy. However, the problem is not in the identification but it is in the estimation approach you are using.

Instead, you can use the g-computation procedure. After estimating the model (as done before), you would now set both $E1$ and $C$ then generate the predicted values. Therefore, the problem is not in identification (daggity is correct in saying the causal effects are identifiable) but is a problem in the estimation strategy (since it is a special case that doesn't apply in the latter).

From a graphical perspective. The $C \rightarrow E1$ in the second DAG doesn't mean much. In our intervention (on both exposures), we are setting both $E1$ and $C$. By setting both, $C$ would no longer be predictive of $E1$ (e.g., we are setting $E1 = 1$ under our intervention and it doesn't depend on the value of $C$).