4
$\begingroup$

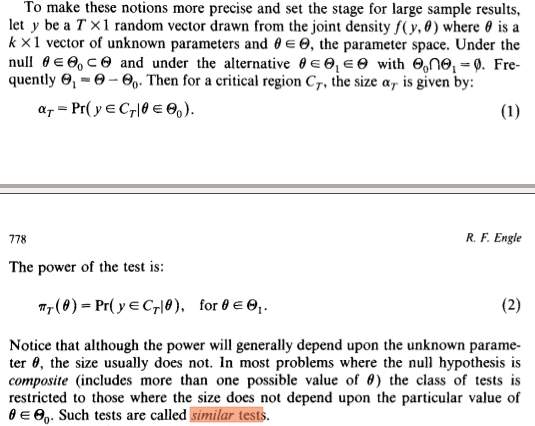
From Wald. Likelihood ratio, and lagrange multiplier tests in econometrics by Robert f. Engle:

(Tests whose) size does not depend upon the particular value of $\theta \in$ null $\Theta_0$ are called similar tests.

I don't quite understand the definition. Isn't the size of a test the sup of false positive rate over null $\Theta_0$? So it doesn't depend on a single $\theta \in \Theta_0$, but on $\Theta_0$?

Following provides more context from the source. Thanks and regards!

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ +1 You have indeed uncovered an abuse of notation: $\alpha_T$ is explicitly an (ill-defined) function of $\Theta_0$ while "$\theta$" enters as a bound variable and so does not even exist semantically. It is clear, though, that Engle is referring at the end to a modified definition of size, equal to $\alpha_T(\theta)=\Pr(y\in C_T\mid \theta)$, which is a function of $\theta$, and that he really wants to define $\alpha_T=\sup_{\theta\in\Theta_0}\alpha_T(\theta)$. $\endgroup$
    – whuber ♦
    Commented Nov 17, 2014 at 18:18

1 Answer 1

Reset to default
3
$\begingroup$

As defined above in Engle's book, there is no supremum in the expression for the size, so it may indeed depend on particular parameter value $\theta \in \Theta_0.$ As far as I know, it is now more common to call this expression null rejection probability (NRP).

The (finite sample) size is then defined as the supremum over the null set $Sz_n=\sup_{\theta \in \Theta_0} Pr(y \in C_T \vert \theta)$ and, furthermore, the asymptotic size is defined as $AsySz = \limsup_{n \to \infty} Sz_n$, the notions you probably messed up the above notion of NRP with.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ This is a good interpretation but it's not what's in the book: $\alpha_T$ is explicitly written to be a function of $\Theta_0$--but that function doesn't even make sense unless $\Pr(y\in C_T|\theta)$ does not vary for $\theta\in\Theta_0$. $\endgroup$
    – whuber ♦
    Commented Nov 17, 2014 at 18:18

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.