How to verify the convergence rate in Monte Carlo simulation?

Question

Given a iid random samples $X\sim N(\theta,1)$, we have a unknown parameter $\theta$ and its estimator $T_n=T_n(X_1,\dots,X_n)$. If we have strictly proved that the convergence rate is $$ |T_n-\theta|=O_p(N^{-1/3}) $$ It seems that $T_n$ is a consistent estimator.

I would like to verify this convergence rate in the Monte Carlo simulation. I'm a little unclear on which quantity should I plot the Monte Carlo estimate? Is it Mean square error MSE? Say I have 1000 samples and each sample is repeated 500 times. It can be drawn that the MSE gradually converges to the x-axis as the number of samples increases.

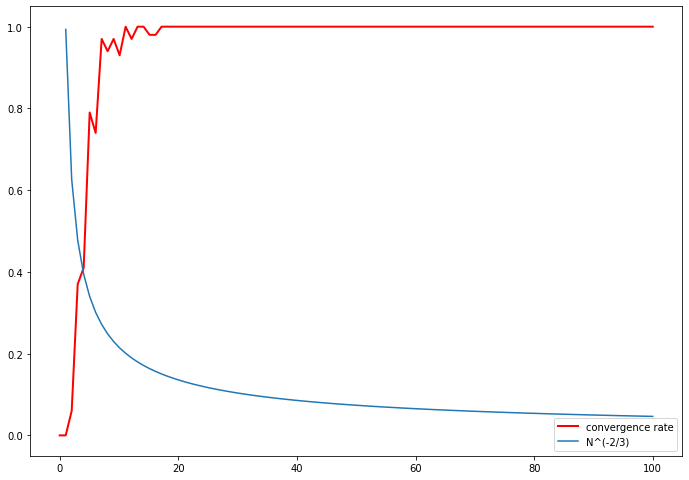
I try to simulate the for a fix $\epsilon>0$, $$ P(|T_n-\theta|>\epsilon) $$

I fix $\epsilon =0.01$ and sample size $n=1000$. That is the mean of $|T_n^{j}-\theta|>\epsilon$ for replications $j=1,\dots, 30$. But I got the following result.

Answer 1

I'm not sure if it will work 100%, but to show that your estimator has the convergence rate of $O_p(N^{-\frac{1}{3}})$ and you want assess it, from the definition for any given $\epsilon > 0$: $$\lim_{n \rightarrow \infty} Pr(|T_n - \theta| > \epsilon) = 0 $$ So to evaluate this visualize I would suggest fixing some small $\epsilon$ and for each $n$ simulate $|T_n - \theta|$ several times, and calculate the empirical $Pr(|T_n - \theta| > \epsilon) $ and plot it. And with this you can see how the convergence will behave against $N^{-\frac{1}{3}}$. BTW it isn't prove anything, you need to make the theoretical calculations to demonstrate any statement.