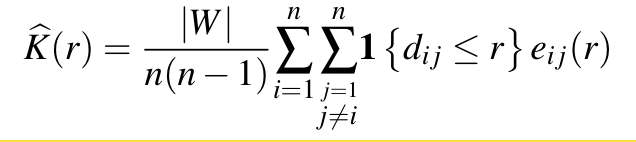Considering the Ripley's K function as used in spatial point-process analysis, or the closely related L function, I am wondering what the limit of the function is as r approaches infinity. I am aware that the function is not well defined as r increases, but I am stuck on how to go about computing this limit, if it exists. My hunch, just based on intuition, is that the function will continue to increase towards infinity. Any input is appreciated. Thank you.
I have provided the equation for the empirical K function below, as it appears in "Spatial Point Patterns: Methodology and Applications with R" by Baddeley, Rubak, and Turner.