"Impossible" crossing of Kaplan-Meier curves

Question

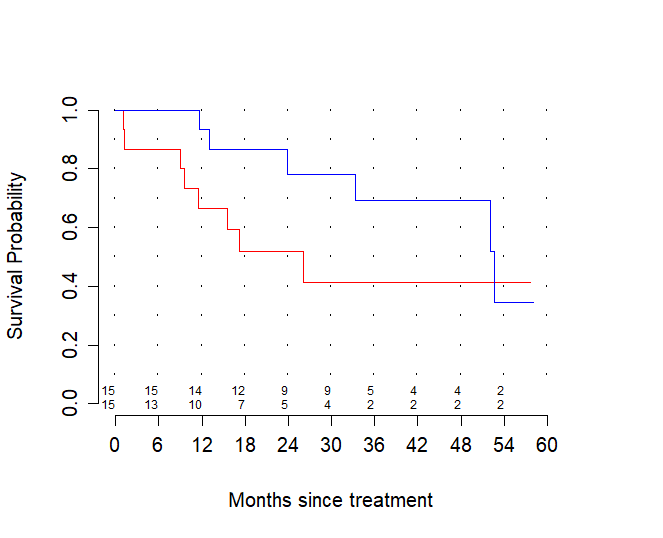
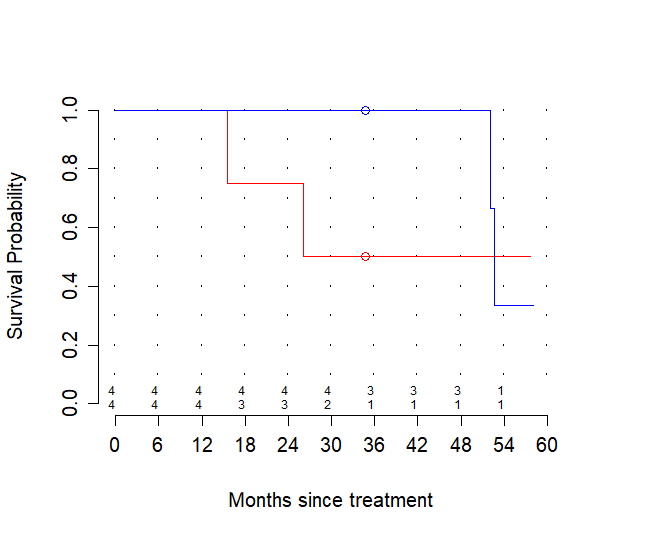
Here is a picture from a small set of lung cancer patients. The blue curve represent overall survival: time from radiotherapy to death of any cause. The red line represents disease free survival: time from radiotherapy to recurrence of disease or death ("whatever comes first", as people tend to write). Both are made with the Kaplan-Meier method, censoring people who had neither event ($n = 7$), or, in the blue curve, had disease recurrence but did no die ($n = 2$), at their last day known to be alive.

Of course normally one would never plot these two curves in one figure because it suggests a comparison between two groups while in reality both curves are talking about the same 15 patients. However I put them in one figure here to make clear that something happens that feels like it should be impossible: the blue curve (overall survival) drops below the red curve (recurrence free survival).

To be clear: for the 'true' curves, to which these KM-curves are just clunky approximations, this is impossible because every OS-event is also an RFS event. At every point in time the percentage of patients still alive should be at least as high as the percentage of patients alive without disease. This is not just a matter of biology but of simple logic.

So what is going on here?

(I figured out the answer, see below, but the reason to post it here is that for once the error is not in my code. The fact that this can happen is an actual weird quirk of the Kaplan-Meier method. Having this example on the internet might save others in the same situation some time searching for an error on their side.)

Answer 1

So the below picture, with only 4 patients from the same dataset explains it all, I believe.

Among the 4 patients there are two that have a recurrence and then, later, die. We recognize them in the jumps in the red curve and then later, more to the right, as the two jumps in the blue curve.

The other two patients are censored. One of them at the very end of the curve, and one of them at the time indicated by the small circles, so after the other two patients had their recurrence, but before these other two patients died.

Now we can see that the KM-curves do indeed do what they always do: the red curve jumps to 3/4 around 13 months since at that point 1/4th of the patients at risk had an event (recurrence). Later, at 26 months it jumps to 1/2 because 1/3rd of the patients then at risk had and event and 2/3 of 3/4 is 1/2.

For the remainder of the available time (i.e. until the censoring of the last patient close to 5 years) the curve stays at 1/2 because nothing happens.

IN the blue curve we have at 52 months that the curve jumps to 2/3 because one of the three patients still at risk at that time dies, and quickly after that jumps to 1/3 because 1 of the 2 patients still at risk died as well and 1/3 is 1/2 of 2/3.

The weird crossing stems from the fact that this 1/3 is less than the height (1/2) of the red curve.

What we see is that indeed the KM-method just does what it always does. There is no programming error, but the sensible behavior of this approximation method can sometimes lead to these unfortunate results

Answer 2

This is a very small sample size to start off with. However, even if that were not the case, as the risk set gets smaller the Kaplan-Meier estimate becomes increasingly unstable (you might even say "unreliable") and there's even recommendations to cut-off (or ignore what happens) the curve after the risk set drops below 10 to 15% of what you originally had.

The KM curve a non-parametric estimate of the survival curve that makes few assumptions about the event time distribution (assumptions are mostly with respect to censoring), which in some sense is desirable (few assumptions) but comes at the cost of more noisy estimates than if you assumed e.g. some parametric survival distribution (or made some assumptions that the hazard rate can't change too crazily over time, as you could e.g. do with some suitable spline approach).

So, when you see the KM curve do big jumps when very few patients are still at risk, this will often be just an effect of it becoming a rather noisy esitmate at that point and this will often just be noise one will at least severely discount (and often pretty much ignore - of course with suitable caution, after all there could be something real here). One thing that can already help put things into perspective are confidence intervals (either for each of the two curves or for the difference between them), that would in this case probably (I'm guessing, but it seems likely) that there is no clear evidence that the true underlying survival curves really cross and that the observed data is also consistent with the curves being decently separated by, say, 0.2 or so.