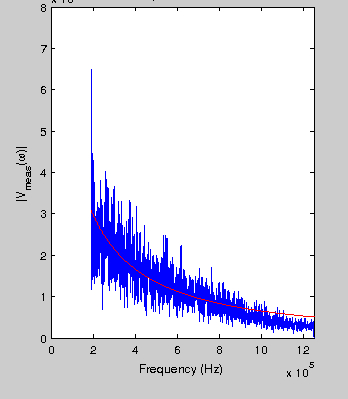
I am fitting a model in the frequency domain, and my fit looks as follows:
As you can see, the model function does not fit the data perfectly, especially in the higher frequencies. So, I examined the residuals and found that a polynomial of the first degree is the missing term in the model function.
What concerns me more is that variance of the data decreases with frequency. What implications does that have on my analysis?
(It seems that looking at the autocorrelation of the residuals also doesn't prove very useful.)
Any ideas would be very helpful. [1]: https://i.sstatic.net/RzrNz.jpg