The leverage is $h_{ii}=\frac{1}{n}+\frac{(x_i-\bar{x})^2}{\sum (x_i-\bar{x})^2}\,$.
The term $\frac{1}{n}$ and the denominator of the second term $\sum (x_i-\bar{x})^2$ are the same for every $i$, so the point with the largest $(x_i-\bar{x})^2$ has the highest leverage.
This means that the point furthest from the mean has the highest leverage.
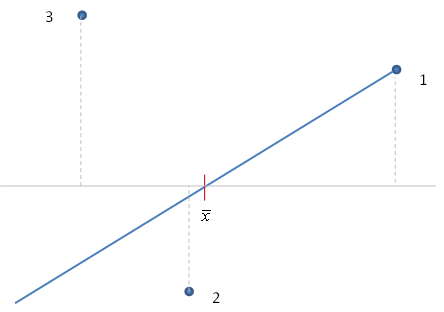
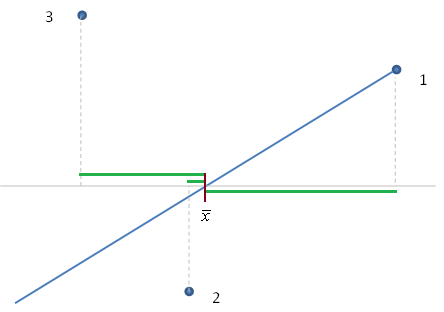
In the diagram, point 1 is the furthest from $\bar x$ in the x-direction, so it will have the largest leverage of the three points.
