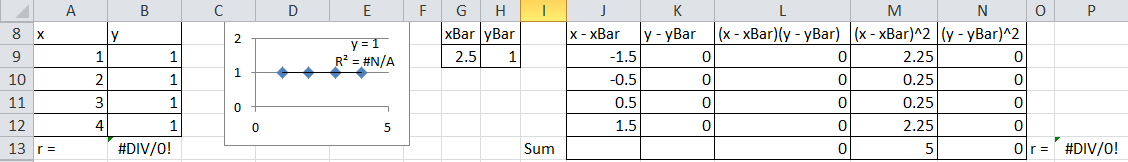
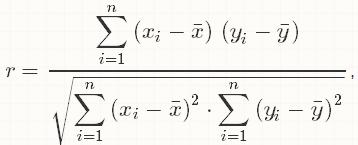- If we use the formula: $$r = \displaystyle \frac{\displaystyle\sum_{i=1}^n \left( x_i - \bar{x} \right) \left( y_i - \bar{y} \right)}{\sqrt{\displaystyle \sum_{i=1}^n \left( x_i - \bar{x}\right)^2 \cdot \sum_{i=1}^n \left( y_i - \bar{y}\right)^2}}$$ for calculating $r$, we get $r = \frac{0}{0}$.
- If we see $r$ as a measurement of the strength and nature of the linear relationship between two variables, we get $r = 0$ because there is no linear relationship in this case.
- If a perfect positive fit gives $r = 1$, and a perfect negative fit gives $r = -1$, then it seems like we should get $r = 1$ or $-1$. (I think this is wrong though.)
$$r = \displaystyle \frac{\displaystyle\sum_{i=1}^n \left( x_i - \bar{x} \right) \left( y_i - \bar{y} \right)}{\sqrt{\displaystyle \sum_{i=1}^n \left( x_i - \bar{x}\right)^2 \cdot \sum_{i=1}^n \left( y_i - \bar{y}\right)^2}}$$
for calculating $r$ we get $r = \frac{0}{0}$.
If we see $r$ as a measurement of the strength and nature of the linear relationship between two variables, we get $r = 0$ because there is no linear relationship in this case.
If a perfect positive fit gives $r = 1$ and a perfect negative fit gives $r = -1$, then it seems like we should get $r = 1$ or $-1$. (I think this is wrong though.)
Which is correct?