A good presentation of a Transfer Function (TF) is here Transfer function in forecasting models - interpretation and alternatively here http://en.wikipedia.org/wiki/Distributed_lag. Since we both have a $Y$ and one $X$ for simplicity sake then I believe that one can form a TF with appropriate assumed lags and appropriate assumed differences of these two series that would match the assumed ECM, illustrating that the ECM is a particular constrained subset of a TF model. Perhaps some other readers (heavy econometricians) have already gone thought the proof/algebra but I will consider your positive suggestion in helping other readers.
After a brief search on the web http://springschool.politics.ox.ac.uk/archive/2008/OxfordECM.pdf discussed how an ECM was a particular case of an ADL (Autoregressive Distributed Lag Model also known as a PDL). An ADL/PDL model is a particular case of a Transfer Function. This material from the above reference shows the equivalence of an ADL and ECM. Note that Transfer Functions are more general than ADL models as they allow explicit decay structure.
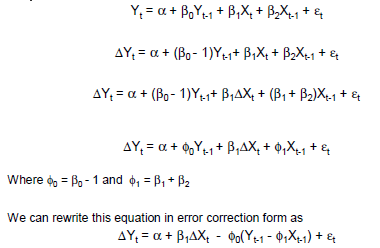
My point is that the powerful model identification features available with Transfer Functions should be used rather than assuming a model because it fits the desire to have simple explanations such as Short Run/Long Run etc. The Transfer Function model/approach enables robustification by allowing the identification of an arbitrary ARIMA component and the detection of Gaussian Violations such as Pulses/Level Shifts/Seasonal Pulses (Seasonal Dummies) and Local Time Trends along with variance/parameter change augmentations.
I would be interested in seeing examples of an ECM that were not functionally equivalent to an ADL model and couldn't be recast as a Transfer Function.
 is an excerpt De Boef and Keele (slide 89 )
is an excerpt De Boef and Keele (slide 89 )
