This is most clearly answered by considering the (cumulative) distribution function.
Specifying the minimum, maximum and the three quartiles specifies exactly 5 points on the cdf, but the cdf between those points may be any monotonic nondecreasing function in between that still passes through those points:
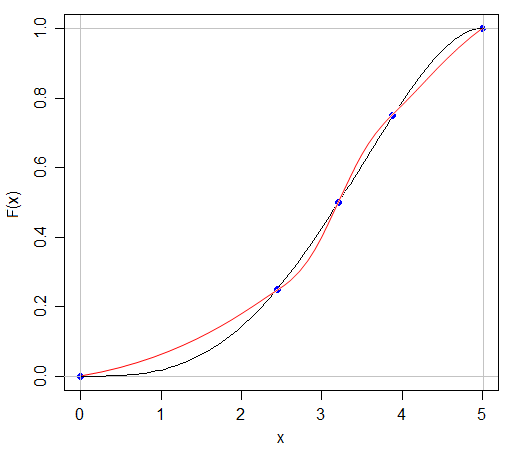
In the drawing, both the red and black CDFs share the same minimum, maximum, and quartiles, but are clearly different distributions. Clearly any number of other CDFs could be specified that also pass through the same five points.
In fact, all we've done is restrict our distribution function to lie within four boxes:
$\qquad$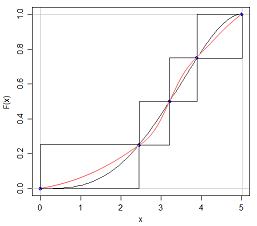
(as long as it also continues to satisfy the other conditions for a CDF). That isn't all that much of a restriction.
The same notion can be applied to sample quantities - two different empirical CDFs may nevertheless have the same five-number summary.
On that subject, see the four examples near the end of this answer, which all have the same five-number summaries, but which have very different looking histograms (which I'll reproduce below):
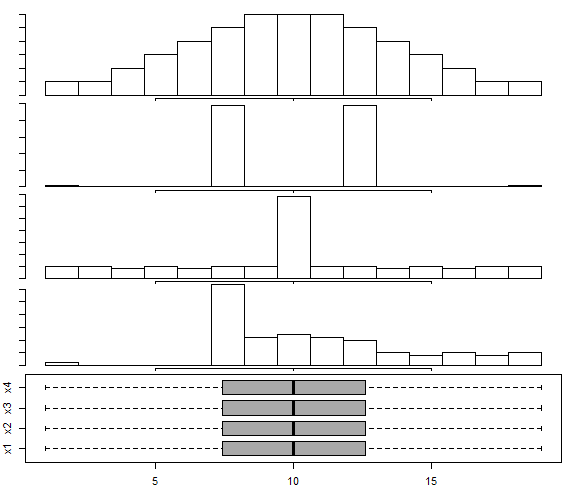
This again emphasizes that five number summaries don't generally do very much to tell us about shape.
