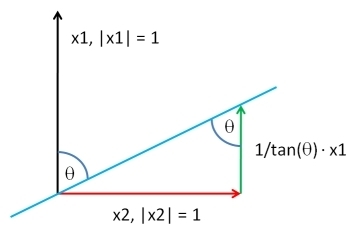
- get fixed vector $x_1$ and a random vector $x_2$
- center both vectors (mean 0), giving vectors $\dot{x}_{1}$, $\dot{x}_{2}$
- make $\dot{x}_{2}$ orthogonal to $\dot{x}_{1}$ (projection onto orthogonal subspace), giving $\dot{x}_{2}^{\perp}$
- scale $\dot{x}_{1}$ and $\dot{x}_{2}^{\perp}$ to length 1, giving $\bar{x}_{1}$ and $\bar{x}_{2}^{\perp}$
- $\bar{x}_{2}^{\perp} + (1/\tan(\theta)) \cdot \bar{x}_{1}$ is the vector whose angle to $\bar{x}_{1}$ is $\theta$, and whose correlation with $\bar{x}_{1}$ thus is $r$. This is also the correlation to $x_1$ since linear transformations leave the correlation unchanged.
a) get fixed vector $x_1$ and a random vector $x_2$ b) center both vectors (mean 0), giving vectors $\dot{x}_{1}$, $\dot{x}_{2}$ c) make $\dot{x}_{2}$ orthogonal to $\dot{x}_{1}$ (projection onto orthogonal subspace), giving $\dot{x}_{2}^{\perp}$ d) scale $\dot{x}_{1}$ and $\dot{x}_{2}^{\perp}$ to length 1, giving $\bar{x}_{1}$ and $\bar{x}_{2}^{\perp}$ e) $\bar{x}_{2}^{\perp} + (1/\tan(\theta)) \cdot \bar{x}_{1}$ is the vector whose angle to $\bar{x}_{1}$ is $\theta$, and whose correlation with $\bar{x}_{1}$ thus is $r$. ThisHere is also the correlation to $x_1$ since linear transformations leave the correlation unchanged.code: