Auto-regressive models (ARIMA) use previous values as predictors depending upon the form of the model and forecasts are adaptive in form generally responding to previous values. Models using time as a predictor can be understood as using previous values to estimate the model parameters (thus previous values do come into play ) but they are otherwise not part of the forecast equation thus being generally non-adaptive or fixed until re-estimation occurs. Models using time or time-squared or time-cubed etc. are anachronistic and generally not used/preferred except in very simple textbooks and in very simple classroom exercises. Models using time variables will generally exhibit auto-correlated residuals thus should be studiously avoided as the presumed model. However my work usually includes/investigates both procedures as tentative/possible approaches since only the data knows which approach is better or which approach delivers a more efficient model.
Response to comment b @Veneeth :
I didn't say less accurate I wrote (implied) different. A time based model predicts based upon the input variable/series 1,2,3,3,...t which means that the prediction for t+2 ,t+ 3 , t+ 4 is fixed or deterministic or unchanged because when you observe y(t+1) as it was before you observed y(t+1). The new value has no effect on the prediction if you don't re-estimate parameters while a model that uses the value of y(t+1) et. al. and is ARIMA based will provide different forecasts. If you use the time predictor approach and re-estimate with y(t+1) in addition to all the previous y's the impact of the new observation will be normally minimal on the model coefficients unless the sample size is very small or the new observation is an anomaly which should be identified and neutralized.
Since @Veneeth asked for a quantitative example , I attempt here to answer that.
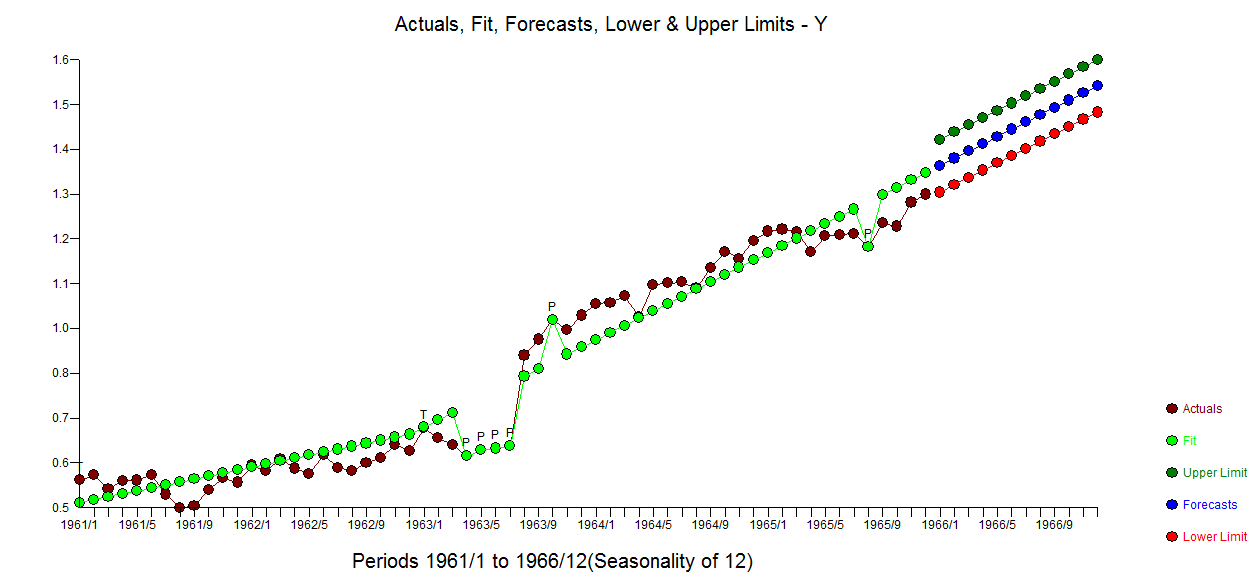
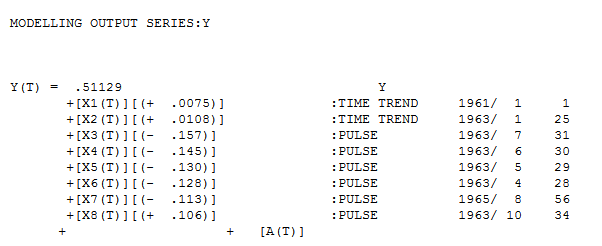
With apologies to Charles Dickens one could entitle this as " A tale of three approaches" I selected a real world example not a trivial textbook example which emphasizes the impact of presumption when it comes to model identification . Consider 1) The time based model (the only non-automatic run ) . Here is the actual fit and forecast 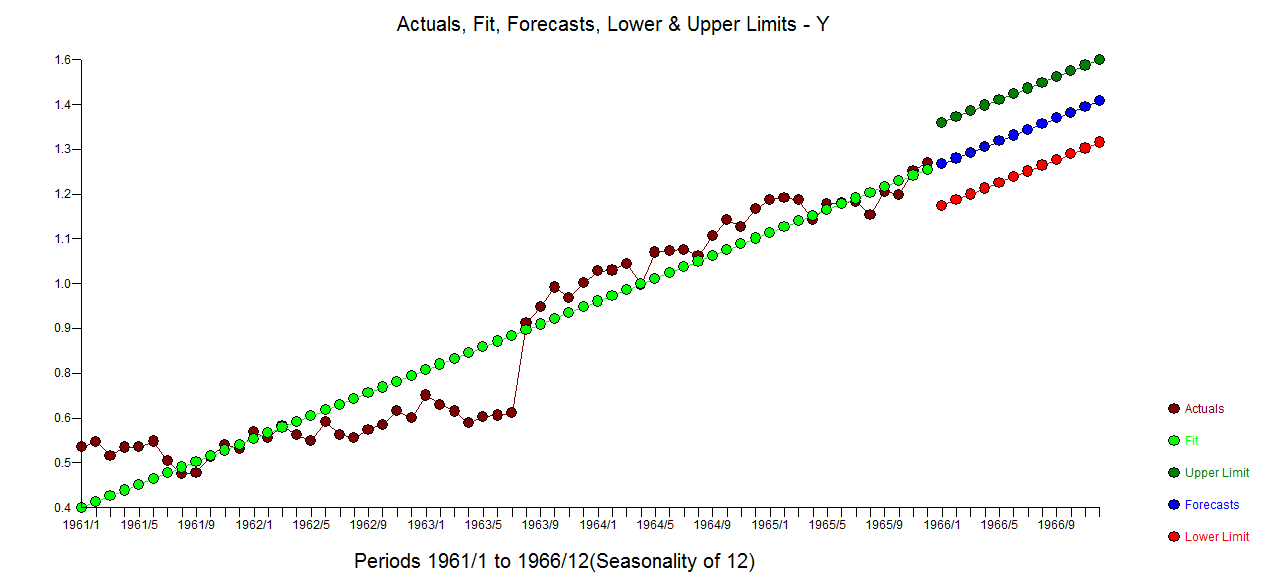 with equation
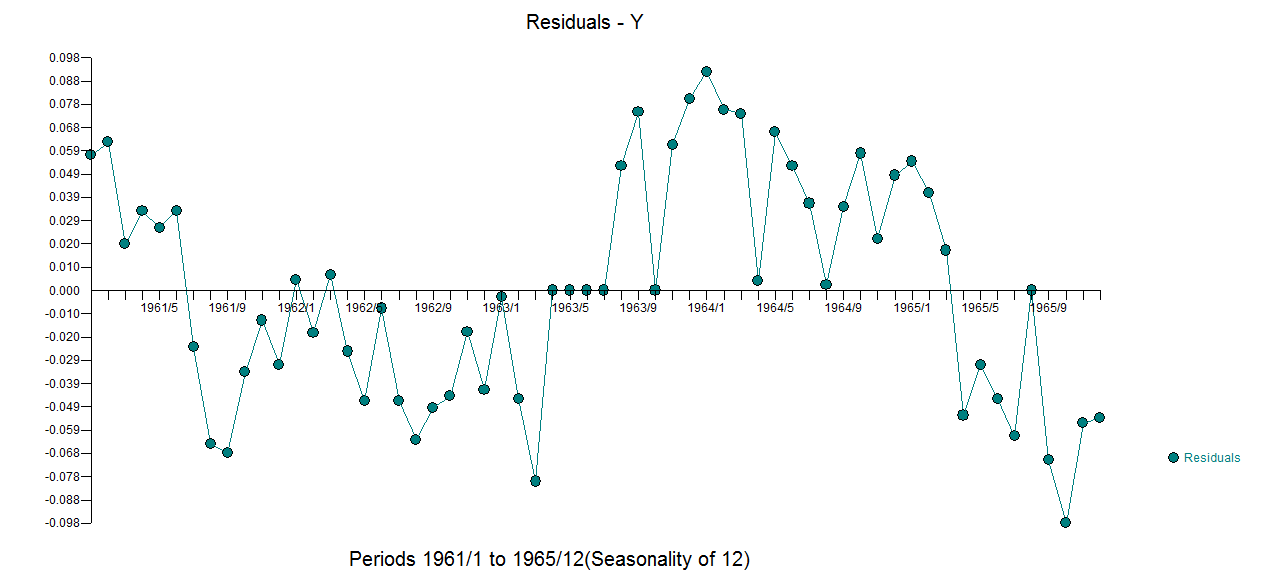
with equation  and residual plot
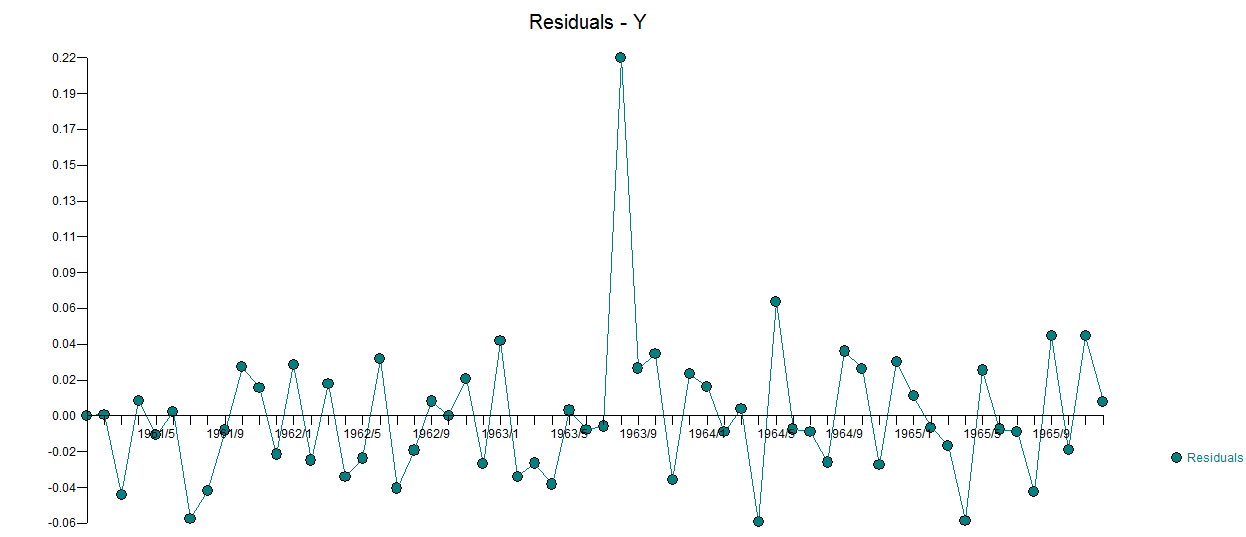
and residual plot  . Followed by 2) The ARIMA model .
. Followed by 2) The ARIMA model . 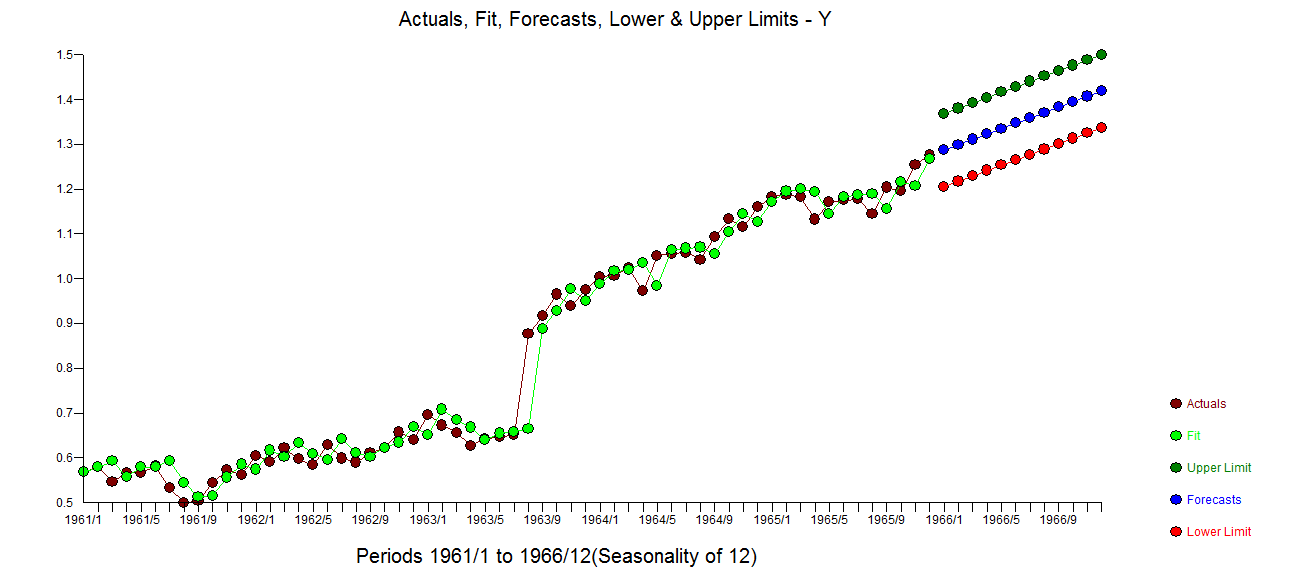

 Now consider a hybrid model incorporating both deterministic structure (input series) and ARIMA
Now consider a hybrid model incorporating both deterministic structure (input series) and ARIMA 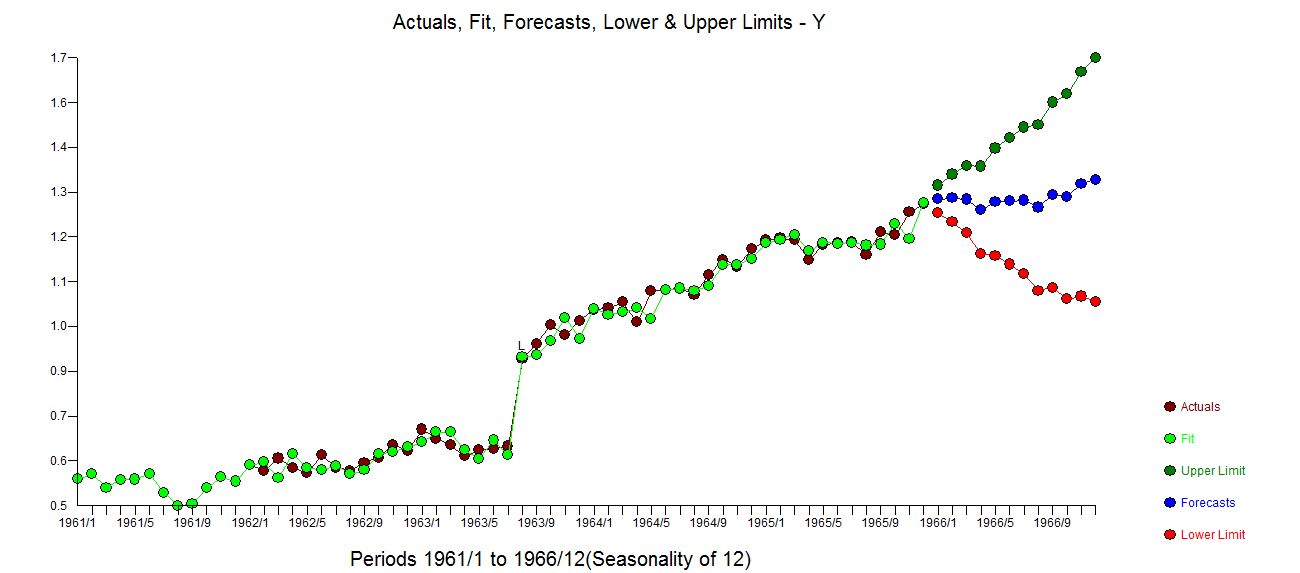

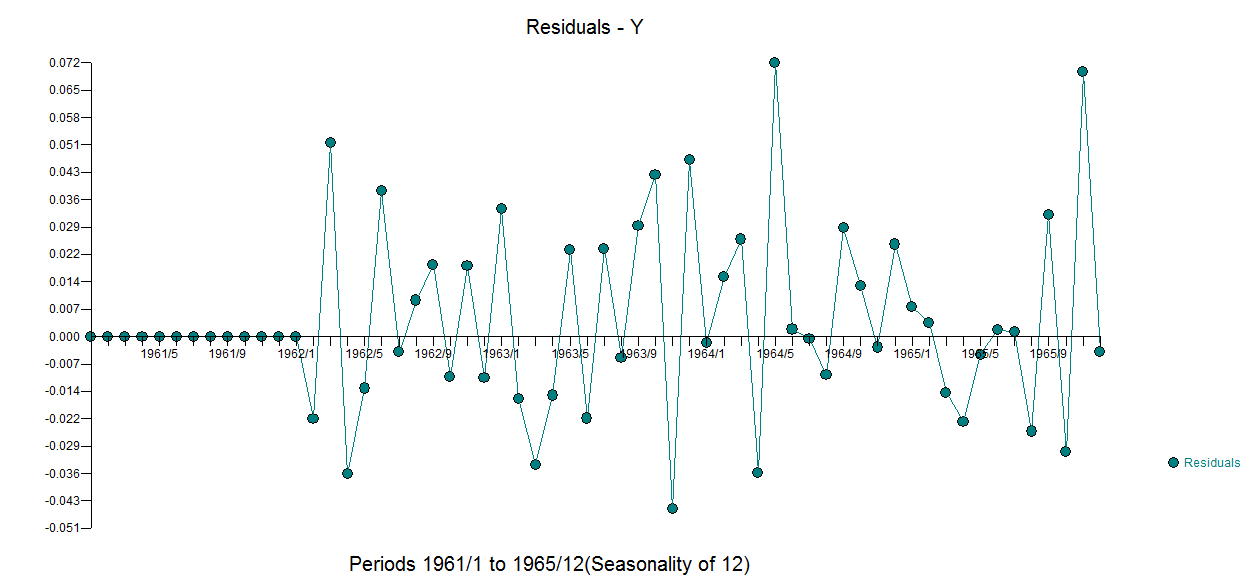 . The variance of the errors from each of the three models reduced dramatically. The deterministic structure that was identified in the hybrid approach was a Level/Step Shift which reflects an intercept change. Visually one could make a case for a possible two-trended model using approach 1 yielding but no no avail
. The variance of the errors from each of the three models reduced dramatically. The deterministic structure that was identified in the hybrid approach was a Level/Step Shift which reflects an intercept change. Visually one could make a case for a possible two-trended model using approach 1 yielding but no no avail