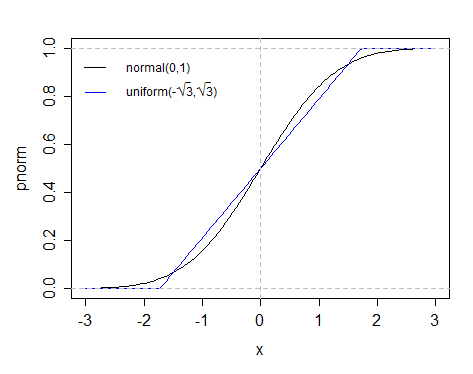
That this is not the case can easily be seen simply by computing the cdf for a different distribution than the normal.
So for example, consider a standard normal (i.e. let's fix $\mu$ and $\sigma$ at 0 and 1, without loss of generality$^\dagger$).
Let's try a uniform on $(-\sqrt{3},\sqrt{3})$ (which also has mean 0 and sd 1) for comparison:
We can see that it's not the case that $\Phi\leq F$ everywhere. There are places where either will exceed the other.
$\dagger$ we can do the same for the general $\mu,\sigma$ case by linearly scaling this example.
Indeed, if you're going to have the means be equal and you're going to make $F\gt\Phi$ in some region, it's going to have to be greatersmaller somewhere else.