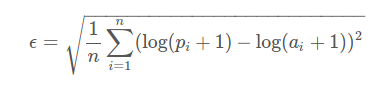To check the performance of a split, as you mentioned MSE and RMSE are the popular approaches.
RMSLE penalizes an under-predicted estimate greater than an over-predicted estimate
ϵ is the RMSLE value (score) n is the total number of observations in the (public/private) data set, pi is your prediction, and ai is the actual response for i. log(x) is the natural logarithm of x.
Ref:
https://www.kaggle.com/wiki/RootMeanSquaredLogarithmicError http://stats.stackexchange.com/a/110610/86202https://stats.stackexchange.com/a/110610/86202