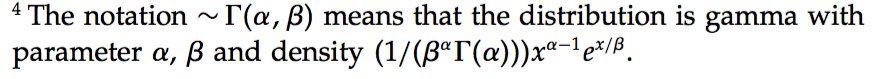I have come upon different parameterizations of the Gamma Distribution, but not with regard to shape-scale or shape-rate. It is rather about the sign in the exponent.
Wolfram lists the pdf as being proportional to $$x^{a-1} \exp{-\frac{x}{b}}$$ https://reference.wolfram.com/language/ref/GammaDistribution.html
However, I saw some papers where the minus sign is missing such that,
$$x^{a-1}\exp{\frac{x}{b}}$$ From my understanding, both parameters $a$ and $b$ have to be positive so this must make some kind of difference. Do I have some error in reasoning here?
broken image fixed (click 'rendered output' or 'side-by-side' to see the difference); for more info, see https://gist.github.com/Glorfindel83/9d954d34385d2ac2597bbe864466259f
Glorfindel
- 1.1k
- 2
- 12
- 18