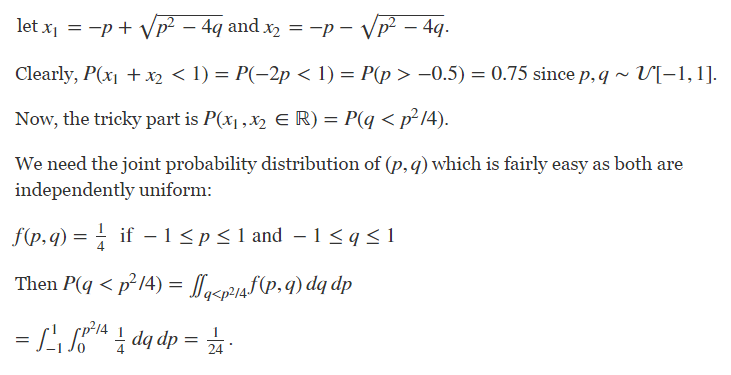We draw two points $p$ and $q$ at random from the interval $[−1, 1]$.
Let $x_1$ and $x_2$ denote the roots of the equation $x^2 + px + q = 0$.
Find the probability that
(a), $x_1, x_2 \in \mathbb{R}$,
(b) $x_1 + x_2 < 1$.
Given that, $x^2 + px + q = 0$, $\Delta = p^2-4q$.
(a)
$x_1, x_2 \in \Bbb R \Rightarrow \Delta \ge 0$
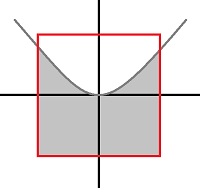
$\Omega = [-1, 1]^2 = \{(p,q) \in \Bbb R^2: p,q \in [-1,1]\}$
$A$ = roots exist.
$A = \{(p,q) \in [-1,1]^2 : p^2 -4q \ge 0 \}$
$x^2 - 4y \ge 0$
$y \le \frac{x^2}{4}$
$\Bbb P(A) = \frac{area-under-the-parabola}{4} = \frac{2 + 2 \cdot half-of-parabola }{4}$
$= \frac{1}{2} + \frac{1}{2} \cdot \int_{0}^{1}\frac{x^2}{4}\, dx$
$ = \frac{13}{24}$
I have several questions regarding the aforementioned solution,
How did the author of the solution know that the parabola passing through the center of the axes and is situated at the positive side of the x-axis?
How was the area between the half of the parabola and the x axis calculated?
What was the mistake with this solution?