To give you an idea of your unfortunate suggested model your ma(1) coefficient is -.98 (nearly -1.0) effectively cancelling your mis-specified differencing operator. I took your 105 values into AUTOBOX , a piece of time series software that I have helped to develop, and (using a slightly different estimation algorithm) and assumed your model . I obtained a coefficient of 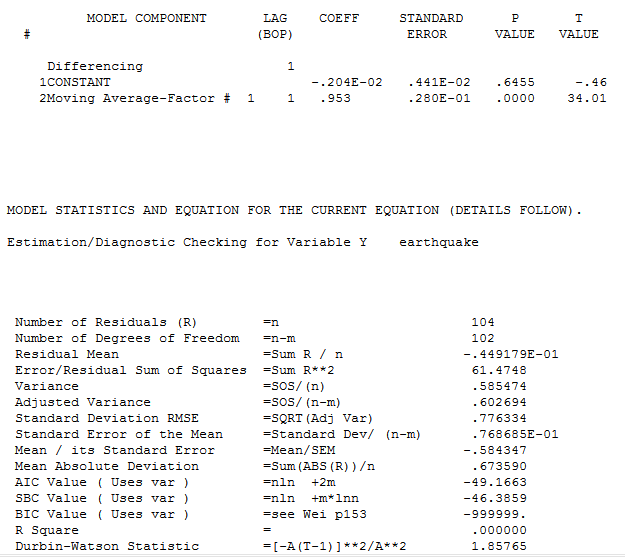 -.953 and an R-Square of 0.0 reflecting the redundancy. Curiously your software reported an R-Square of .457 which motivated some research on my part. If one unnecessarilly differences a series then one is injecting structure and the new series will have greater variance Variance of difference of $x_{i,t}$ and $x_{i,t+1}$Variance of difference of $x_{i,t}$ and $x_{i,t+1}$ . If one then models this inflated series with an MA(1) then one can then get an R Squared of .457 .This reporting of the R Squared of the differenced series as compared to the R squared of the observed series is very bad statistics in my opinion and represents an inability to deal holistically with the observed series and the ARMA struture. Software is just as good as the last time it was checked . This is poor practice and defintely non-standard. There is nonsense and there is nonsense but the most nonsensical of them all is statistical nonsense.
-.953 and an R-Square of 0.0 reflecting the redundancy. Curiously your software reported an R-Square of .457 which motivated some research on my part. If one unnecessarilly differences a series then one is injecting structure and the new series will have greater variance Variance of difference of $x_{i,t}$ and $x_{i,t+1}$Variance of difference of $x_{i,t}$ and $x_{i,t+1}$ . If one then models this inflated series with an MA(1) then one can then get an R Squared of .457 .This reporting of the R Squared of the differenced series as compared to the R squared of the observed series is very bad statistics in my opinion and represents an inability to deal holistically with the observed series and the ARMA struture. Software is just as good as the last time it was checked . This is poor practice and defintely non-standard. There is nonsense and there is nonsense but the most nonsensical of them all is statistical nonsense.
replaced http://stats.stackexchange.com/ with https://stats.stackexchange.com/
