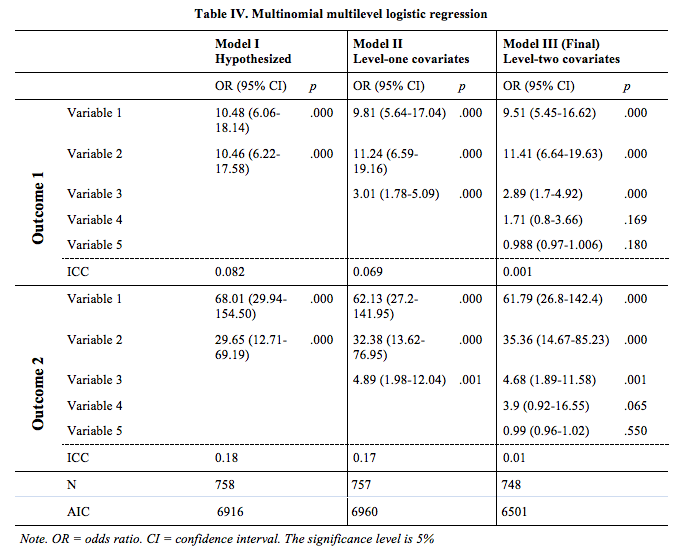
I am using a multilevel regression model with a multinomial outcome (i.e., three unordered categories) as dependent variable, assigned the numeric values 0, 1, and 2. 0 being the reference category.
The The model can be seen below:
My question is: Is it possible to compare the odds ratio effect size for Variable 1 on both outcomes when having an multinomial dependent variabe? I have seen people deriving an odds ratio by dividing them (e.g., 61.79 / 9.51 = 6.49 OR, Final model), meaning that having Outcome 2 is 6.49 times the odds higher than having Outcome 1, when one is exposed to Variable 1, compared to the reference category.
Is this legitimate? I simply can't find any literature backing this up.
Hope you all can help.