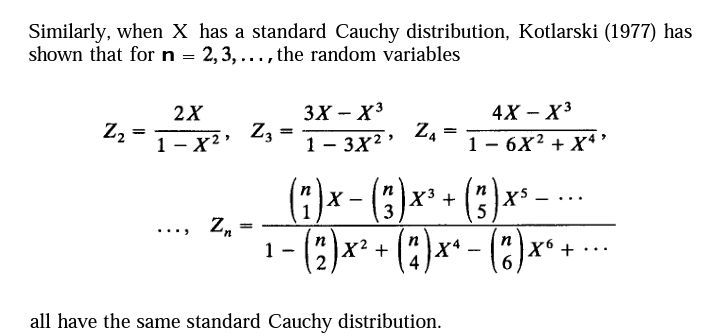
If $X\sim\mathcal C(0,1)$, find the distribution of $Y=\frac{2X}{1-X^2}$.
We have $F_Y(y)=\mathrm{Pr}(Y\le y)$
$\qquad\qquad\qquad=\mathrm{Pr}\left(\frac{2X}{1-X^2}\le y\right)$
$\qquad\qquad=\begin{cases} \mathrm{Pr}\left(X\in\left(-\infty,\frac{-1-\sqrt{1+y^2}}{y}\right]\right)+\mathrm{Pr}\left(X\in\left(-1,\frac{-1+\sqrt{1+y^2}}{y}\right]\right),\text{if}\quad y>0\\ \mathrm{Pr}\left(X\in\left(-1,\frac{-1+\sqrt{1+y^2}}{y}\right]\right)+\mathrm{Pr}\left(X\in\left(1,\frac{-1-\sqrt{1+y^2}}{y}\right]\right),\text{if}\quad y<0 \end{cases}$
I wonder if the above case distinction is correct or not.
On the other hand, the following seems a simpler method:
We can write $Y=\tan(2\tan^{-1}X)$ using the identity $\frac{2\tan z}{1-\tan^2z}=\tan 2z$
Now, $X\sim\mathcal C(0,1)\implies\tan^{-1}X\sim\mathcal R\left(-\frac{\pi}{2},\frac{\pi}{2}\right)$
$\qquad\qquad\qquad\quad\implies 2\tan^{-1}X\sim\mathcal R(-\pi,\pi)$
$\qquad\qquad\qquad\quad\implies\tan\left(2\tan^{-1}X\right)\sim\mathcal C(0,1)$, the last one being a 2-to-1 transformation.
But if I am asked to derive the distribution of $Y$ from definition, I guess the first method is how I should proceed. The calculation becomes a bit messy, but do I reach the correct conclusion? Any alternate solution is also welcome.
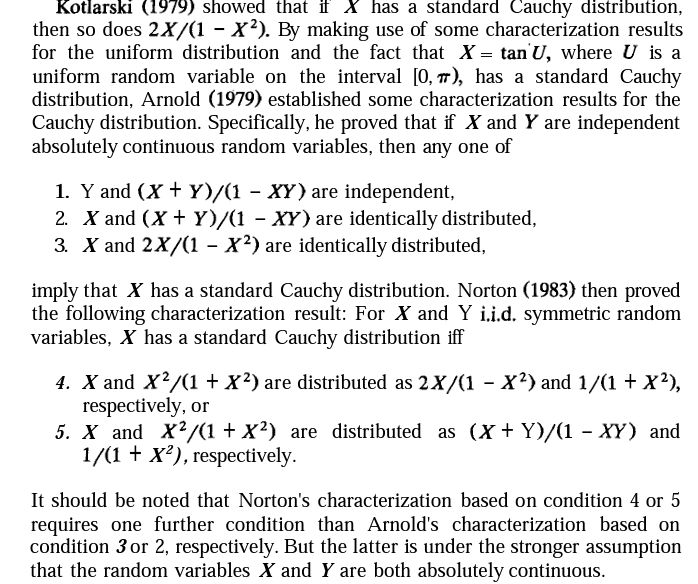
Continuous Univariate Distributions (Vol.1) by Johnson-Kotz-Balakrishnan has highlighted this property of the Cauchy distribution. As it turns out, this is just a special case of a general result.