It seems that on some its subrange your dependent variable is constant or is exactly linearly dependent on the predictor(s). Let's have two correlated variables, X and Y (Y is dependent). The scatterplot is on the left.
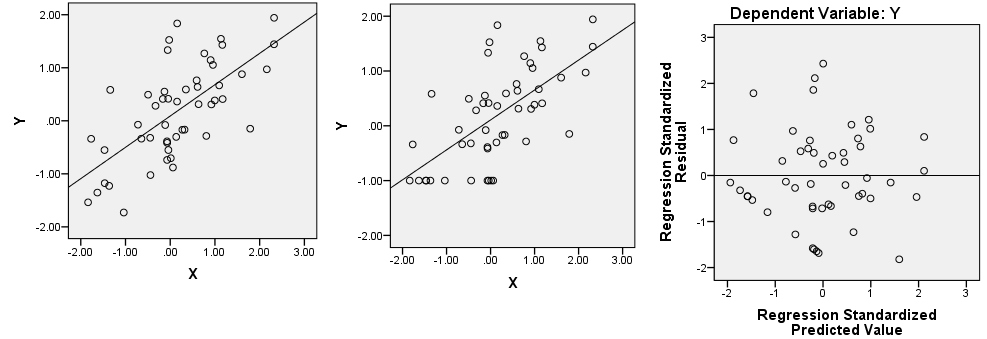
Let's return, as example, on the first ("constant") possibility. Recode all Y values from lowest to -0.5 to a single value -1 (see picture in the centre). Regress Y on X and plot residuals scatter, that is, rotate the central picture so that the prediction line is horizontal now. Does it resemble your picture?
