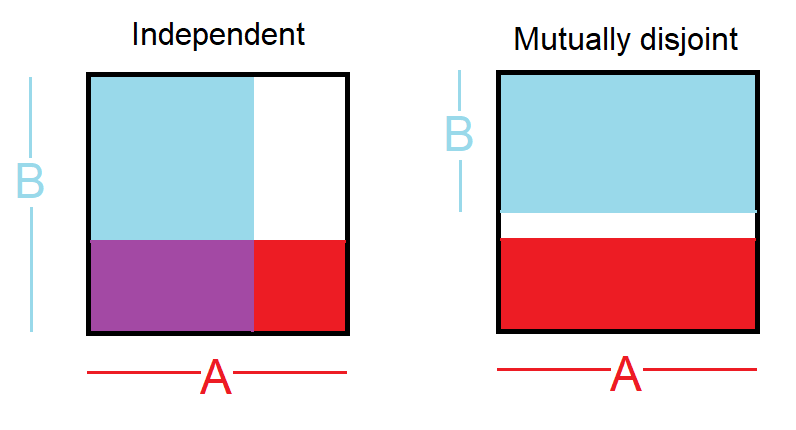
There is a difference between
- independent events: $\mathbb P(A \cap B) =\mathbb P(A)\,\mathbb P(B)$, i.e. $\mathbb P(A \mid B)= \mathbb P(A)$ so knowing one happened gives no information about whether the other happened
- mutually disjoint events: $\mathbb P(A \cap B) = 0$, i.e. $\mathbb P(A \mid B)= 0$ so knowing one happened means the other almost certainly did not happen
You asked for a picture. This might help: