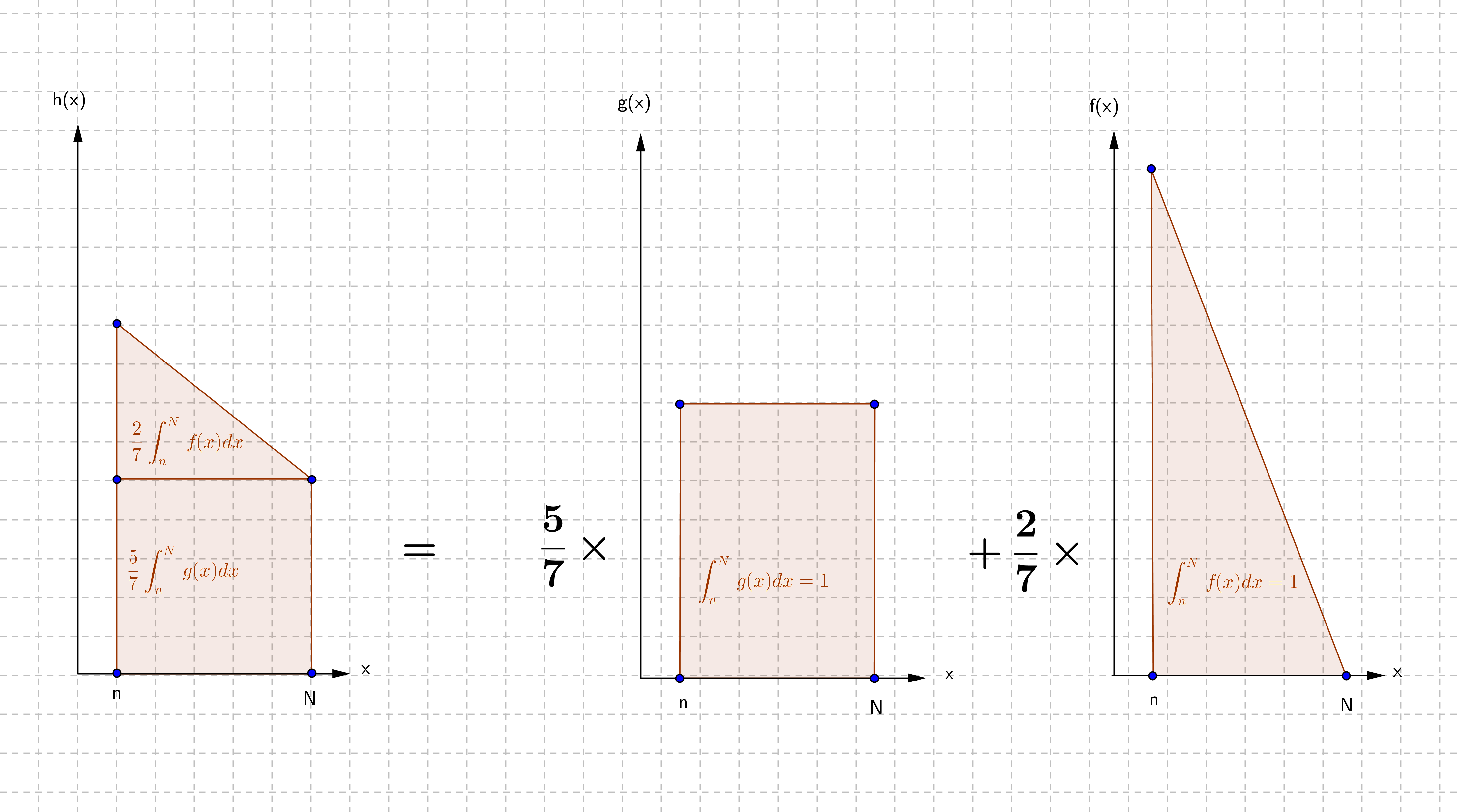
Your image shows the sum of two functions which relates to a mixture distribution:
$$h(x) = a g(x) + (1-a) f(x)$$
(see also this discussion)
with
- the continuous distribution:
$$g(x) = \begin{cases} \frac{1}{N-n} & \quad \text{ for $ n \leq x\leq$ N } \\ 0 & \quad \text{otherwise}\end{cases}$$
- a triangular distribution:
$$f(x) = \begin{cases} 2 \frac{N-x}{(N-n)^2} & \quad \text{ for $ n \leq x\leq$ N } \\ 0 & \quad \text{otherwise}\end{cases}$$
You do not need to worry about the constant of integration since:
$$\begin{array}{rcl} \int_n^N h(x)dx &=& \int_n^N \underbrace{( a g(x) + (1-a) f(x))}_{=h(x)} dx \\ & = & \int_n^N a g(x) dx + \int_n^N (1-a) f(x) dx \\ & = & a \underbrace{\int_n^N g(x) dx}_{=1} + (1-a) \underbrace{\int_n^N f(x) dx}_{=1} \\ & = & a + (1-a) = 1 \end{array} $$
To get your figure you need to add 5/7 times the uniform (squarerectangular) distribution and 2/7 times the triangle distribution.
$$h(x) = \frac{5}{7} g(x) + \frac{2}{7} f(x) = \begin{cases} \frac{\frac{5}{7} + \frac{4}{7} \frac{N-x}{N-n} }{N-n} & \quad \text{ for $ n \leq x\leq$ N } \\ 0 & \quad \text{otherwise}\end{cases}$$