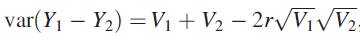I know that the variance of the difference of two correlated variables, $Y_1$ and $Y_2$ , is what the below formula shows, requiring $r$ the correlation between the two variables.
But suppose I have an amply large random sample of each of above variables, $Y_1^*$ and $Y_2^*$, in isolation.
Does the variance of $Y_2^* - Y_1^*$ give me a good estimate of the variance of $Y_2 -Y_2$?
Note: In this formula $V_1$ and $V_2$ are the variances of $Y_1$ and $Y_2$, respectively.