Comment. A surprisingly broad question.
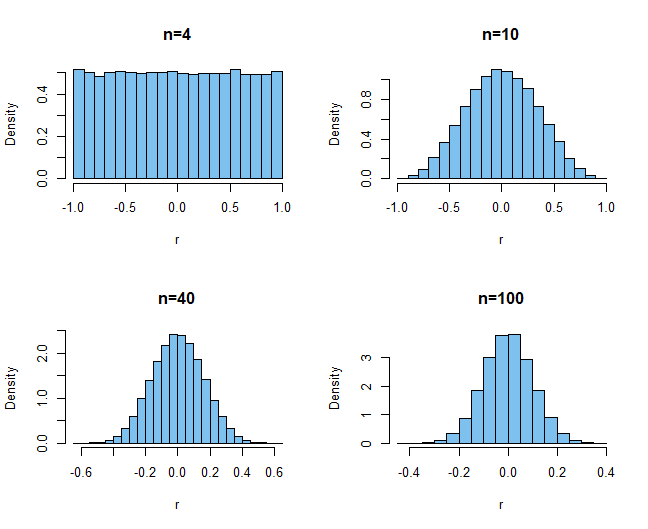
This page shows simulation results for correlations of two independent standard uniform distributions. Results seem to differ greatly for various (especially small) numbers $n$ of pairs of points. Each histogram represents $100,000$ values of $r.$
For normal data, of course, there is Fisher's work on the distribution of $r.$ (See Wikipedia.)
When you say 'average correlation', do you mean $E(r)$ or $E(|r|)?$ When you say 'maximum correlation', what sample sizes do you have in mind to keep the maximum below 1? To start: I hope you can specify a few cases of greatest interest (dimensions, sample sizes, uniform vs. normal vs. what other distributions?).
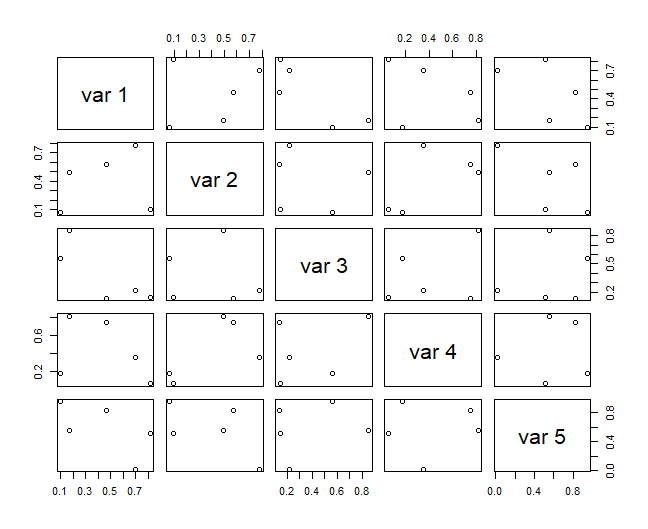
Addendum per @whuber's Comment: Further experimentation. Five observations on each of five independent uniform variables.
set.seed(906)
n = 5; m = 5; u = runif(n*m)
DTA = matrix(u, nrow=m)
cor(DTA)
[,1] [,2] [,3] [,4] [,5]
[1,] 1.0000000 0.1957409 -0.8233160 -0.39093048 -0.62212314
[2,] 0.1957409 1.0000000 -0.1255770 0.62306226 -0.61205725
[3,] -0.8233160 -0.1255770 1.0000000 0.36625813 0.22424637
[4,] -0.3909305 0.6230623 0.3662581 1.00000000 0.08519501
[5,] -0.6221231 -0.6120572 0.2242464 0.08519501 1.00000000
max(abs(cor(DTA)-diag(5)))
[1] 0.823316
Highest absolute correlation happens to be about $|r| \approx 0.82,$ between
variables 1 and 3. Matrix plot shows all ${5 \choose 2}$ pairs---see center top row. (AbsoluteMax absolute correlations this high are not rare. Tried several runs before
this one with set.seed to post.)
pairs(DTA)