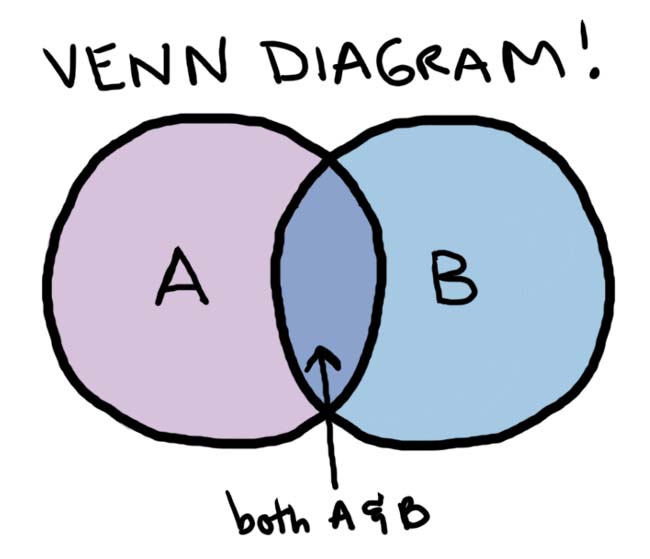
The simplest way to understand conditional probability at an introductory level is to undertake exercises where you relate probabilities to areas and relative-areas in a Venn diagram. There are many resources available that teach conditional probability in this way, but I will give a simple outline here. Consider a Venn diagram with two events $A$ and $B$ represented as interlocking circles (picture taken from this related question), and suppose we treat the areas as being equivalent to the probabilities. The probability of $A$ is the area of the purple circle and the probability of $B$ is the area of the blue circle. The conditional probability of $A$ given $B$ would be the relative size of the intersection where both these events occur (the dark-blue area in the middle) relative to the area of the blue circle.
If you do a quick search online you will find hundreds of introductory resources that explain conditional probability by reference to Venn diagrams, and this gives you a nice visual illustration of the concept. You will also be able to find exercises to test your knowledge. I would recommend this as a starting point for anyone having difficulty understanding the relationship between conditional probability and the AND/orOR operations betweenon events.