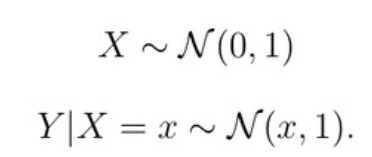 $$X \sim \mathcal{N}(0,1)$$
$$Y|X = x \sim \mathcal{N}(x,1)$$
$$X \sim \mathcal{N}(0,1)$$
$$Y|X = x \sim \mathcal{N}(x,1)$$
I'm familiar with the normal use of tilde in indicating a random variable follows a distribution (such as in the first line of the attached image), but I haven't run into the tilde as it is used in the second line of the attached image. Is this saying that the random variable is itself the mean? That doesn't make much sense to me.
