Return to Answer

An important property of the variance is that it is a natural way to describe the spread of a distribution. It is the second moment of a distribution and its definition has a direct connection to the moment of inertia considered in physics.

We can use this connection to give an entertaining way to give a feeling of what variance is and and how to distinguish variance and the average spread of a distribution.

Suppose you have a sample $x_i, i = 1, ..., n$ and a large rigid stick with negligible weight. There is a scale on this stick in cm. On this stick, you allocate weights of 1 kg at the distances that correspond to the deviations $\bar{x} - x_i$ of the individual values $x_i$ from their mean $\bar{x}$. You balance the stick on a pivot point precisely on its center of mass so that it can rotate freely in a horizontal plane. The center of mass of all weights is located at the point on the scale that corresponds to the average of your sample. Then its variance is equal to the moment of inertia or angular mass of the stick, up to a constant. That is, how much the stick resists rotation.

Here comes the difference to the Mean Average Error/ Deviation or MAE. For the MAE it is equivalent to have 10 values (or 10 kg) $x_i$ at a distance $\bar{x} - x_i = 10 $ cm from the mean and having one value $x_i$ or 1 kg at a distance 100 cm from the mean. However, the variance (the inertia of the stick) is much larger if you place one weight at a distance of 100 cm from the mean (and pivot point) than when placing a weight of 10 at a distance 10 from the mean.

An important property of the variance is that it is a natural way to describe the spread of a distribution. It is the second moment of a distribution and its definition has a direct connection to the moment of inertia considered in physics.

We can use this connection to give an entertaining impression of what variance is.

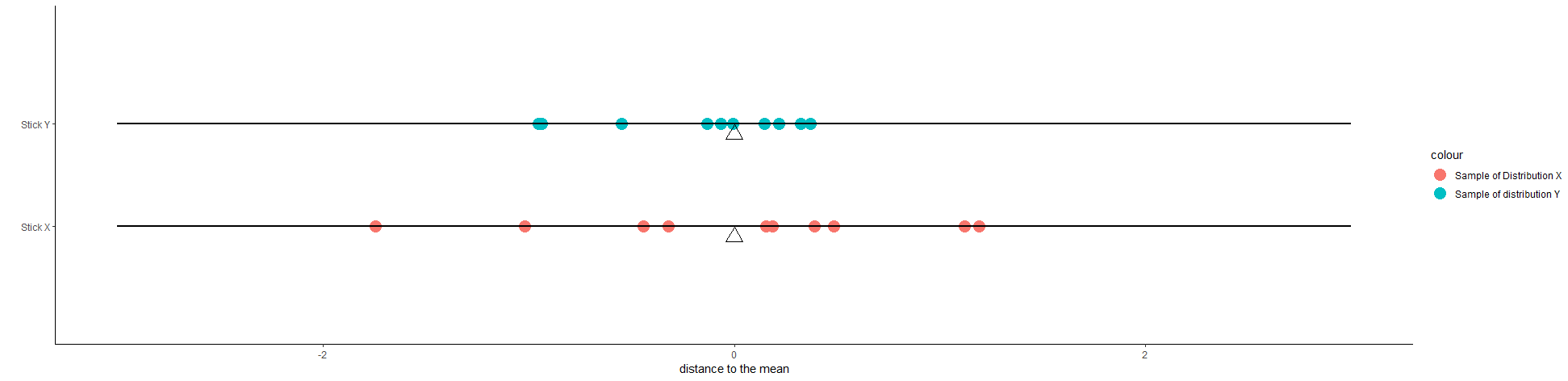
Suppose you have two distributions $X$ and $Y$ and you want to compare how spread out they are in terms of the variance. So you take a sample of each distribution $x_i, y_i, i = 1, ..., n$ and two large rigid sticks with negligible weight. On each stick you place a weight of 1 kg for each of your values $x_i$ and $y_i$ so that the $x_i$ and $y_i$ are placed on separate sticks. You allocate weights of 1 kg around the mid point of the sticks, such that the distance between the midpoint of each stick and the weight correspond to the deviations $\bar{x} - x_i$, $\bar{y} - y_i$ of the individual values $x_i, y_i$ from their respective mean $\bar{x}$ and $\bar{y}$. Then you balance these stick on pivot points precisely on their mid points which is their center of mass by construction. So each stick can rotate freely in a horizontal plane.

The variances of $X$ and $Y$ are equal to the moments of inertia or angular mass of the weighted sticks. That is, roughly speaking how much each stick resists to changes of its rotation.

So we can compare the variance of the sticks as follows.

Suppose they are rotating at the same speed and you were set up to play jump or get hit.

The stick that hits you harder corresponds to the distribution of higher variance.

This picture might be used to remember why higher variance can correspond to higher risk (when comparing distribution of stock returns for example)

This illustration can highlight the difference between the standard deviation and the average distance to the mean (or Mean Average Error/ deviation or MAE). For the MAE it is equivalent to have 10 values (or 10 kg) $x_i$ at a distance $\bar{x} - x_i = 1 $ m from the mean and having one value $x_i$ or 1 kg at a distance 10 m from the mean. However, the standard deviation (the square root of the inertia of the stick) is much larger if you place one weight at a distance of 10 m from the mean (and pivot point) than when placing a weight of 10 kg at a distance of 1m from the mean.

Imagine you could choose the stick to play jump or get hit with but you would see the world through lenses of average absolute distance. Then you would not be able to distinguish risky sticks from less risky sticks.

An important property of the variance is that it is a natural way to describe the spread of a distribution. It is the second moment of a distribution and here is how it is connected to that concept in physics.

Suppose you have a sample $x_i, i = 1, ..., n$ and a large rigid stick with negligible weight. There is a scale on this stick in cm. On this stick, you allocate weights of 1 kg at the distances that correspond to the deviations $\bar{x} - x_i$ of the individual values $x_i$ from their mean $\bar{x}$. You balance the stick on a pivot point precisely on its center of mass so that it can rotate freely in a horizontal plane. The center of mass of all weights is located at the point on the scale that corresponds to the average of your sample. Then its variance is equal to the moment of inertia or angular mass of the stick, up to a constant. That is, how much the stick resists rotation.

Here comes the difference to the Mean Average Error/ Deviation or MAE. For the MAE it is equivalent to have 10 values (or 10 kg) $x_i$ at a distance $\bar{x} - x_i = 10 $ cm from the mean and having one value $x_i$ or 1 kg at a distance 100 cm from the mean. However, the variance (the inertia of the stick) is much larger if you place one weight at a distance of 100 cm from the mean (and pivot point) than when placing a weight of 10 at a distance 10 from the mean.

An important property of the variance is that it is a natural way to describe the spread of a distribution. It is the second moment of a distribution and its definition has a direct connection to the moment of inertia considered in physics.

We can use this connection to give an entertaining way to give a feeling of what variance is and and how to distinguish variance and the average spread of a distribution.

Suppose you have a sample $x_i, i = 1, ..., n$ and a large rigid stick with negligible weight. There is a scale on this stick in cm. On this stick, you allocate weights of 1 kg at the distances that correspond to the deviations $\bar{x} - x_i$ of the individual values $x_i$ from their mean $\bar{x}$. You balance the stick on a pivot point precisely on its center of mass so that it can rotate freely in a horizontal plane. The center of mass of all weights is located at the point on the scale that corresponds to the average of your sample. Then its variance is equal to the moment of inertia or angular mass of the stick, up to a constant. That is, how much the stick resists rotation.

Here comes the difference to the Mean Average Error/ Deviation or MAE. For the MAE it is equivalent to have 10 values (or 10 kg) $x_i$ at a distance $\bar{x} - x_i = 10 $ cm from the mean and having one value $x_i$ or 1 kg at a distance 100 cm from the mean. However, the variance (the inertia of the stick) is much larger if you place one weight at a distance of 100 cm from the mean (and pivot point) than when placing a weight of 10 at a distance 10 from the mean.

An important property of the variance is that it is a natural way to describe the spread of a distribution. It is the second moment of a distribution and here is how it is connected to that concept in physics.

Suppose you have a sample $x_i, i = 1, ..., n$ and a large rigid stick with negligible weight. There is a scale on this stick in cm. On this stick, you allocate weights of 1 kg at the distances that correspond to the deviations $\bar{x} - x_i$ of the individual values $x_i$ from their mean $\bar{x}$. You allocate the stick on a pivot point precisely on its center of mass so that it can rotate freely in a horizontal plane. The center of mass of all weights is located at the point on the scale that corresponds to the average of your sample. Then its variance is equal to the moment of inertia or angular mass of the stick, up to a constant. That is, how much the stick resists rotation.

Here comes the difference to the Mean Average Error/ Deviation or MAE. For the MAE it is equivalent to have 10 values (or 10 kg) $x_i$ at a distance $\bar{x} - x_i = 10 $ cm from the mean and having one value $x_i$ or 1 kg at a distance 100 cm from the mean. However, the variance (the inertia of the stick) is much larger if you place one weight at a distance of 100 cm from the mean (and pivot point) than when placing a weight of 10 at a distance 10 from the mean.

An important property of the variance is that it is a natural way to describe the spread of a distribution. It is the second moment of a distribution and here is how it is connected to that concept in physics.

Suppose you have a sample $x_i, i = 1, ..., n$ and a large rigid stick with negligible weight. There is a scale on this stick in cm. On this stick, you allocate weights of 1 kg at the distances that correspond to the deviations $\bar{x} - x_i$ of the individual values $x_i$ from their mean $\bar{x}$. You balance the stick on a pivot point precisely on its center of mass so that it can rotate freely in a horizontal plane. The center of mass of all weights is located at the point on the scale that corresponds to the average of your sample. Then its variance is equal to the moment of inertia or angular mass of the stick, up to a constant. That is, how much the stick resists rotation.

Here comes the difference to the Mean Average Error/ Deviation or MAE. For the MAE it is equivalent to have 10 values (or 10 kg) $x_i$ at a distance $\bar{x} - x_i = 10 $ cm from the mean and having one value $x_i$ or 1 kg at a distance 100 cm from the mean. However, the variance (the inertia of the stick) is much larger if you place one weight at a distance of 100 cm from the mean (and pivot point) than when placing a weight of 10 at a distance 10 from the mean.