0
$\begingroup$

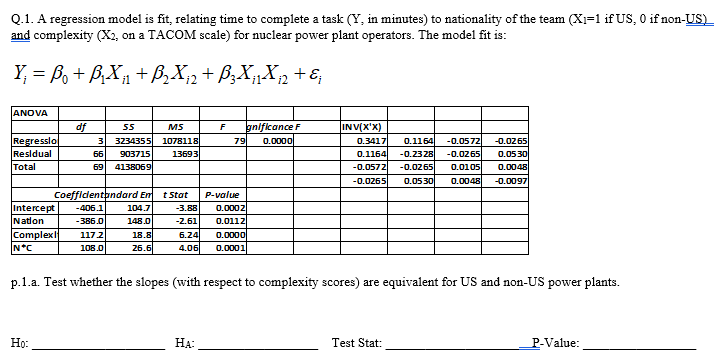
I usually take a look at the diagonal elements of the matrix $(X'X)^{-1}$ and the $MSE$ in order to determine the standard deviation of the regression coefficients. I encountered a problem from a practice test that has a negative entry in the diagonal for the matrix $(X'X)^{-1}$. Can this be possible?

This is the problem enter image description here

I am testing for $\beta_3=0$, which in order to do, I would need to take $\frac{\hat{\beta_3}}{SE(\beta_3)}$, but the negative number in the diagonal of the inverse matrix throws me off.

Any help is much appreciated.

Improve this question
$\endgroup$
3
  • $\begingroup$ Is this a homework? $\endgroup$
    – patagonicus
    Commented Dec 4, 2021 at 1:43
  • $\begingroup$ No, this is an old practice exam, and my only question is about the standard error for the regression coefficient. I use the diagonal element of the inverse matrix provided, but it has a negative entry, so this would not make sense. $\endgroup$
    – Stiven G
    Commented Dec 4, 2021 at 3:15
  • $\begingroup$ This might help stats.stackexchange.com/questions/96327/… $\endgroup$
    – patagonicus
    Commented Dec 4, 2021 at 4:20

1 Answer 1

Reset to default
0
$\begingroup$

$\boldsymbol{X}^{\prime}\boldsymbol{X}$ is positive semi-definite. Hence, for any vector $\boldsymbol{v}$, $\boldsymbol{v}^{\prime}\boldsymbol{X}^{\prime}\boldsymbol{X}\boldsymbol{v} \ge 0$. If we let $\boldsymbol{v}$ denote a standard basis vector, then this inequality implies the diagonal elements must be non-negative. There is a typo in the problem.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.