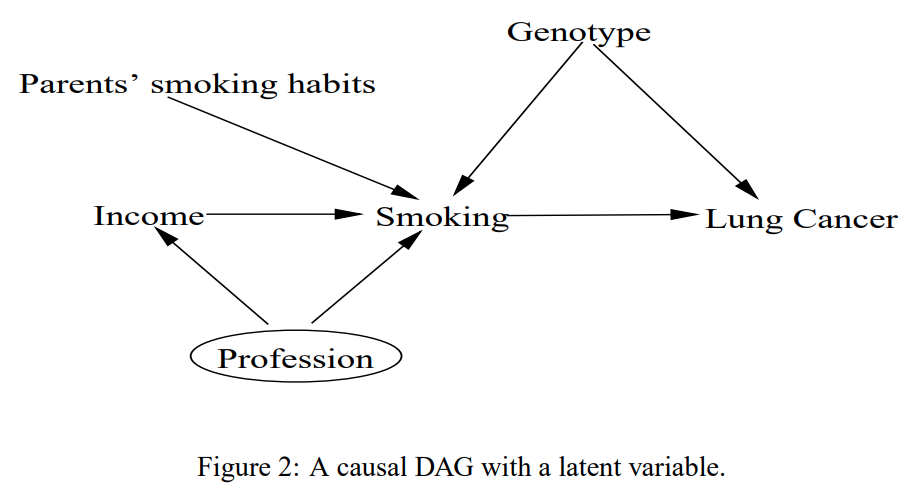
On page 1443 of the linked paper, the authors present the following causal DAG (Directed Acyclic Graph) with a latent variable (Profession).
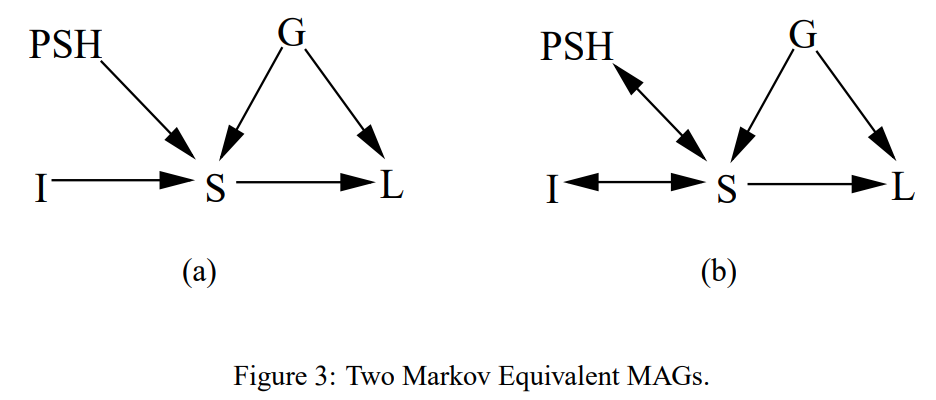
On the following page, they present the 2 MAGs below with the one on the left (a) described as "the causal MAG that corresponds to the causal DAG". The one on the right (b) is presented as a Markov-equivalent MAG to the causal MAG.
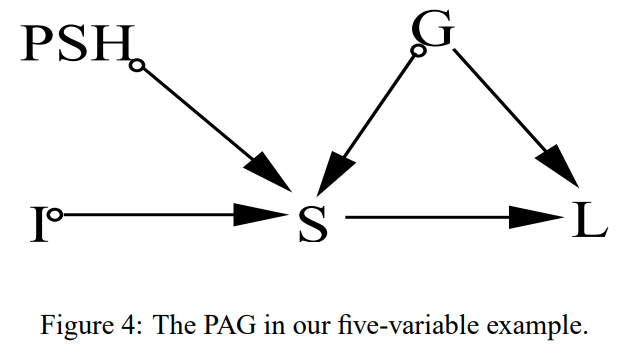
Finally, on page 1445, the authors present the PAG that corresponds to the causal MAG. Please see below.
Can someone help me think through why the 2 MAGs (Maximal Ancestral Graph) are Markov Equivalent (imply the same constraints by the m-separation criterion)? I would also like to convince myself that the MAG on the left is indeed the causal MAG for the causal DAG? How is a MAG is related to a DAG and a PAG to a MAG? As I understood it: a PAG is Markov-equivalent class of MAGs, but a MAG is not a Markov-equivalent class of DAGs (instead a PCDAG is). Is that correct? I ask these questions because I am not yet fully comfortable with all these terms: PAGs, MAG, m-separation, active paths, etc.
Reference: Causal Reasoning with Ancestral Graphs