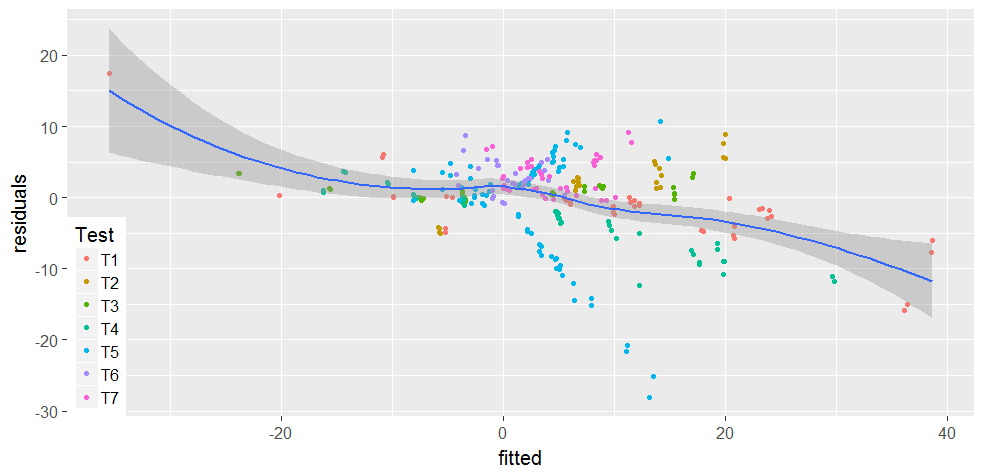
I'm trying to build a regression from the results of a campaign of physical experiments, consisting of 7 different test campaigns. In each test campaign, all test points are repeated two times, once with a vertical excitation and once with an horizontal excitation. The reason is that at the time of the tests, people were unsure about the isotropy of the physical system. The results of a nonlinear regression are (residuals vs fitted plot):
You can clearly see that most residuals go in pairs: in other words, the residual for a test with vertical excitation and the corresponding test with horizontal excitation are very close. Physically, I interpret this as an indication that the system is very close to isotropic (the vertical and the horizontal test give more or less the same result in most cases). Statistically, this means that my data are not iid: thus the confidence intervals returned from this nonlinear regression, which are based on the hypothesis of iid data and on asymptotic normality, will likely underestimate the real variability in my parameters. I can of course repeat the NLS regression excluding the repeated tests:
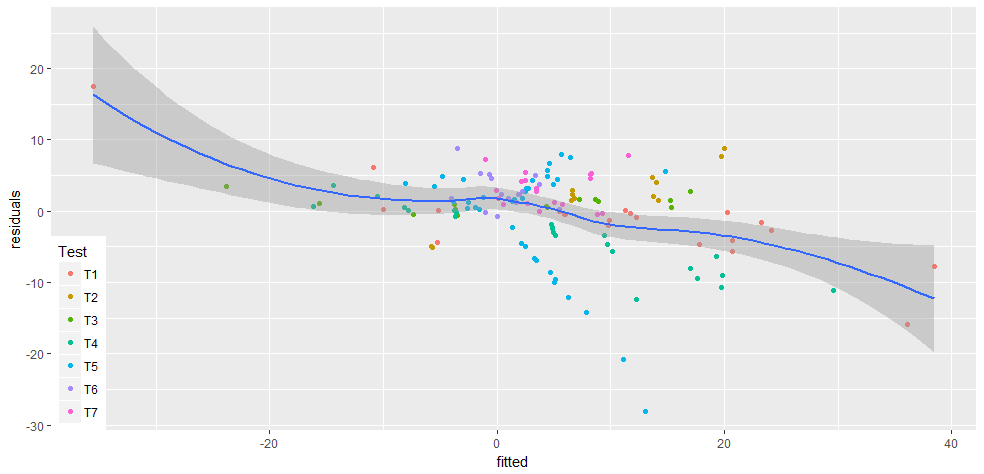
Now I have less points, and the confidence intervals for the estimated parameters are correspondingly larger. I'm not very happy about that. Do I really have to work with half my data points, or could I correct for the limited serial correlation in a less drastic way?