-1
$\begingroup$

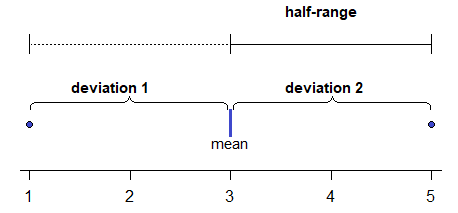
We know that mean deviation and standard deviation are two different things but why are both of them equal to Range/2?

Range ($R$) = Highest value - lowest value

Mean deviation ($\text{MD}$) = average absolute distance from the mean

Standard deviation ($\text{SD}$,$s_n$) $=\sqrt{\frac{\sum_{i=1}^n (x_i-\bar{x})^2}{n}}$

Edit: This is for two unequal observations. My book has a lengthy algebraic proof but I don't follow it.

Can anyone please help me understand this? Is there some shortcut way to show this statement is true?

Improve this question
$\endgroup$
16
  • 2
    $\begingroup$ I think your question could benefit from some clarification. Neither standard deviation nor mean absolute deviation equals $\frac{R(max - min)}{2}$ unless perhaps in some highly artificial constructed scenario (and what is R?). $\endgroup$
    – einar
    Commented Dec 2, 2017 at 17:02
  • 1
    $\begingroup$ The statement in the question is not true in general (take the numbers 0, 1,2 and 5 as a sample and see for yourself). It may be true in some particular situation but you have presented no specific instance that would make it true, so the question makes no sense and must be clarified to be answerable in any way other than the answer you have right now. I have removed your comment under what is a correct answer to the question as posed - if so many people don't understand the question, the problem lies in the question. Please add enough context to the question that we can see the situation. $\endgroup$
    – Glen_b
    Commented Dec 2, 2017 at 22:24
  • 1
    $\begingroup$ You see the part in the top image where it says: "For two unequal observations"? That is what was essential to put in your question. (Why would you choose to leave that critical detail out after multiple requests for clarification?) ... But now I am glad you posted the whole set of fuzzy images -- because now it's clear your question is answered in your book. It contains a complete detailed proof of the assertion for the case of two observations. It shows you, step by step why it's true --- your question is now fully answered in your question. There's nothing left to answer! $\endgroup$
    – Glen_b
    Commented Dec 2, 2017 at 22:59
  • 1
    $\begingroup$ @user55439 The reason you can't delete is because you have a several-times upvoted answer; you don't get to remove other people's contributions. I think there's a reasonable underlying question here, and with some additional effort this could be a well-received question. I have made several edits to get your question closer to a good one. I would put the "two observation" part into the title but I don't want to make the first answer you got look mistaken; this is a reasonable compromise version for the present. Hopefully my explanation is of some help. $\endgroup$
    – Glen_b
    Commented Dec 3, 2017 at 0:57
  • 1
    $\begingroup$ It appears you've had some poorly received questions elsewhere as well, it might be worth comparing the current question to the one you initially posted, and see how it addresses the issues people raised at various times. It may help you figure out the kinds of thing you can do to have questions that get a better reception (as well as an answer you might be able to use). Writing good questions is a difficult skill (more difficult than answering them, I think), but it's an essential part of getting help. $\endgroup$
    – Glen_b
    Commented Dec 3, 2017 at 5:10

2 Answers 2

Reset to default
3
$\begingroup$

Without going to the R(Range)/2 part, why do you think MD = SD? That is NOT generally true (although data can be constructed such that MD = SD).

For example: x = [-10, 0, 10] SD(x) = sqrt((10^2 + 0 + 10^2)/3) = ~2.72 (or = 10 if you devide by N-1 instead of N) MD(x) = (10 + 0 + 10)/3) = 6.66...

SD(x) != MD(x)

Improve this answer
$\endgroup$
0
3
$\begingroup$

They aren't equal in general, as Gomunkul points out; in the two observation case they are; that's the situation your book is referring to.

Since you already have a proof, my best guess is that you're mostly seeking intuition. I can offer a somewhat more intuitive explanation of why this is the case for two values. During the explanation, it helps to think of a concrete example -- let's say the values are 1 and 5.

  • With two values, the mean is the midpoint of the two values. Both values are equidistant from the mean. (3 is the mean, both values are 2 away from that.)

  • range is twice the distance of the midpoint to either of the observations. (The range is 5-1=4, and the distance of the ends to the midpoint is half of that -- i.e. 2.)

diagram of the example points showing midpoint=mean, half-range=mean deviation

  • since both points are equally far from the mean, the mean deviation is that distance of either point from the mean (i.e. half the range). With two points the distance to half-way is always half the distance to the other end. (When both points are 2 away from the middle, the mean deviation is 2, which must be half the range)

  • with standard deviation*, $s_n$, first look at the variance, $s_n^2$. The variance is the average of the squared distances from the mean. Note that each point is the mean deviation away (half the range) from the mean, so when you square those you get that each point contributes $\text{MD}^2$.

    The average of two $\text{MD}^2$ values is also $\text{MD}^2$; so $\text{Var}=\text{MD}^2$. When you take the square root to get back to the standard deviation, you will get the mean deviation. ($(5-3)^2 = 2^2, (1-3)^2=2^2$, both of which are just the square of the distance from the middle to the ends (i.e. the square of MD); the average of $2^2$ and $2^2$ is $2^2$, still the square of the mean deviation)

  • None of this argument depends on the particular values chosen for the example.

* this is the $n$-denominator standard deviation, not the Bessel-corrected version.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Is there any video in YouTube that will help me too ? $\endgroup$
    – user55439
    Commented Dec 3, 2017 at 17:55
  • $\begingroup$ I have no idea. $\endgroup$
    – Glen_b
    Commented Dec 3, 2017 at 21:19

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.