Some questions about the Generalized Method of Moments

Question

Here are three (somewhat related) questions about the (Generalized) Method of Moments. I have only just today started studying this method.

1. Concerning the following statement by Greene:

Why is $g$ not a function only of $Y_t$? My understanding was that $g$ should be computable from the sample, but if the true parameter (which is unknown) influences $g$, then we cannot compute it.

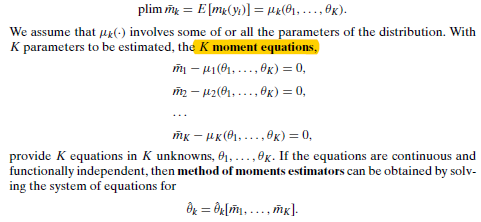
2. Greene states that for the Methods of Moments estimator, we have $E(m_k(y_l))=\mu_k(\theta_1,...,\theta_k)$, where I understand $m_k(\cdot)$ to be the $k$'th sample-moment and $\mu_k$ the $k$'th population moment.

However, Greene then states that for the Generalized methods of moments estimator we need the "moment conditions":

perhaps these $m_{l,K}$ refer to something other than the moments, but I don't understand why this equation is different for the Generalized method, compared to the (non-generalized) method of moments. (Greene doesn't seem to explain this, if I'm not mistaken).

3. This also partially justifies the following question: Is the "moment condition" synonymous with "moment equation"? Greene uses both words, without clearly defining a difference.

Answer 1

1. Note that we are calculating the expected value of the moment function here, not the sample mean of the moment function. The expected value is, necessarily, a function of the parameters. We need to know this function because we, in effect, reverse-engineer the parameter estimates from the sample means of the moment functions by using it.

A trivial example is the MOM estimator of the parameter of an Exponential distribution; we need to know how the parameter relates to the population mean (a very simple $g(y,\theta_0)$), as in, $\mathbb{E}[Y_t |\theta_0] = 1/\theta_0$. We can then use the sample mean and set $\hat{\theta}_0 = 1/\bar{y}$.

2. The $m_{l,K}$ don't have to be the usual central moments, they are "generalized" in the sense of being somewhat arbitrary functions that have expected values rather than just integer powers of $y$ that have expected values (subject to various conditions, of course, that Greene outlines.) For example, for a weighted estimator, you might have $\mathbb{E}[y_t w(y_t)] = 0$, where the $w(y_t)$ downweight extreme values of $y_t$ for robustness purposes. For more examples, see the Scope section of the Wikipedia page.

3. I'm not sure about this, not having Greene at hand, but my take on it is that the "moment conditions" are slightly more general, because you have the possibility of other variables involved, e.g., independent variables in a linear regression. With MOM estimates in their most basic form, you just have a distribution, a bunch of parameters, and a bunch of moments. With GMM, you can make the "bunch of parameters" be parameterized functions of other variables, and you can make the "bunch of moments" be expected values of functions that aren't themselves moments. But this is really just the difference between a special case and the general case, which I tend to think of as a distinction without a difference from a terminology perspective unless there's really something special about the special case.