1
$\begingroup$

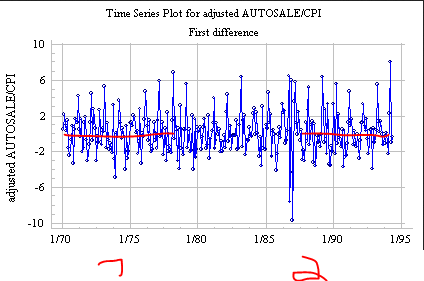
I am extremely puzzled... In textbooks I read that the "stationary property" is having the same statistical properties, in two chunks of my time series.

I do not understand... How can I possibly check that?, Clearly if I do any chunk against all others, that will be infinite possibilities since the chunk can also vary in size.

I can't understand how the TS could for example keep the same mean?

To me, for it to be the same mean always it has to be a straight line, otherwise I could just pick two specific chunks so that it will make my mean different and conclude is not stationary.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
0
$\begingroup$

It means theoretically. In an experimental setup, different chunks of the series will have probably different statistics, even the average values as you suggest. But, for example, if a theoretical analysis shows thata $E[x_t]=\mu_x$, i.e. constant, then the series is said to be mean stationary. This means at any time of the series, the mean of the sample at that time is $\mu_x$. For different chunks of data, you won't get exactly equal means of course; but the theoretical analysis suggest that you could expect approximate means among large enough chunks.

Another example would be autocorrelation, i.e. a second order statistics. If your time series have stationary autocorrelation, then the correlation between two of its samples won't depend on exactly where they are, instead it'll depend on the relative time distance between them, i.e. $r_x(t_1,t_2)=r_x(t_1-t_2)$. This means, even if you analyze first $10^4$ samples or the next, you should be obtaining similar results (within ergodic assumption) because the realization is assumed to come from a 2nd order stationary process.

Improve this answer
$\endgroup$
0
$\begingroup$

To visualize the answer from gunes:

It means theoretically. In an experimental setup, different chunks of the series will have probably different statistics, even the average values as you suggest. But, for example, if a theoretical analysis shows thata E[xt]=μx, i.e. constant, then the series is said to be mean stationary. This means at any time of the series, the mean of the sample at that time is μx. For different chunks of data, you won't get exactly equal means of course; but the theoretical analysis suggest that you could expect approximate means among large enough chunks.

enter image description here

This is a stationary process in two chunks, based on the first difference.

Improve this answer
$\endgroup$
2
  • $\begingroup$ How/who decides which chunks and it's size? Just by finding any two chunks with same mean I can conclude it is stationary? $\endgroup$
    – Chicago1988
    Commented Sep 13, 2019 at 13:37
  • $\begingroup$ no, this is just how this process works in the back $\endgroup$
    – PV8
    Commented Sep 13, 2019 at 13:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.