Show the bivariate normal cdf evaluated at (0,0) is increasing in the correlation coefficient

Question

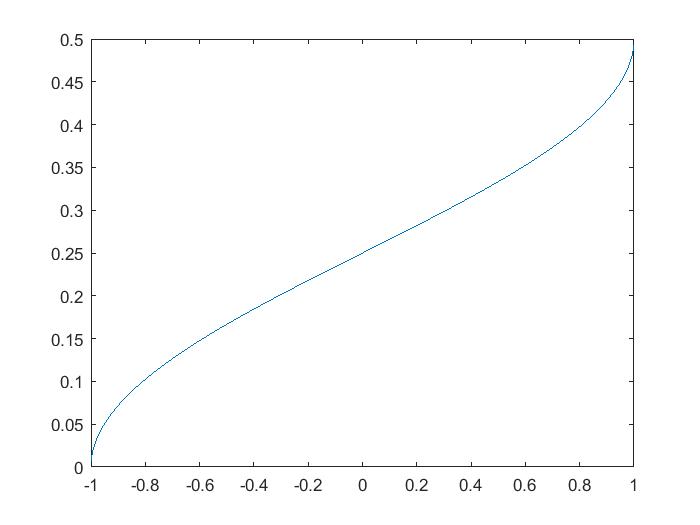
$\begin{bmatrix}\epsilon_{1}\\ \epsilon_{2}\end{bmatrix}\sim N(\begin{bmatrix}0\\0\end{bmatrix},\begin{bmatrix}1,\rho\\ \rho, 1\end{bmatrix})$. Show that the joint cdf evaluated at (0,0), i.e., $F_{\epsilon_{1},\epsilon_{2}}(0,0,\rho)\equiv Pr(\epsilon_{1}\leq 0, \epsilon_{2} \leq0)$ is monotonically increasing in $\rho \in (-1,1)$. Numerical calculation below (using 2000 grid points) shows that this is almost indeed the case. Thanks!

Answer 1

Take independent standard normal random variables $X$ and $Y$. Then the joint distribution of $X$ and $\rho X-\sqrt{1-\rho^2}\,Y$ is the same as joint distribution of $\epsilon_1$, $\epsilon_2$. This is Cholesky's decomposition. Then $$ \mathbb P(\epsilon_1\leq 0, \epsilon_2\leq 0) = \mathbb P\left(X\leq 0, \rho X-\sqrt{1-\rho^2}\,Y \leq 0\right)=\mathbb P\left(X\leq 0, Y\geq \frac{\rho}{\sqrt{1-\rho^2}}X \right) $$ The function $\frac{\rho}{\sqrt{1-\rho^2}}$ is monotonly increasing in $\rho\in[-1,1]$. Therefore, if $\rho_1<\rho_2$ then $\frac{\rho_1}{\sqrt{1-\rho_1^2}}<\frac{\rho_2}{\sqrt{1-\rho_2^2}}$. Then for $X< 0$,
$$ \frac{\rho_1}{\sqrt{1-\rho_1^2}}X > \frac{\rho_2}{\sqrt{1-\rho_2^2}}X $$ and if $Y$ is greater the first, it is definitely greater the second. So we have the inclusion of the events: $$ \left\{X\leq 0, Y\geq \frac{\rho_1}{\sqrt{1-\rho_1^2}}X \right\} \subset \left\{X\leq 0, Y\geq \frac{\rho_2}{\sqrt{1-\rho_2^2}}X \right\}. $$ This events are equal only when $X=0$ which has zero probability. Therefore the probability of the first event is strictly less than the probability of the second, which means that $\mathbb P(\epsilon_1\leq 0, \epsilon_2\leq 0) $ is strictly increasing in $\rho$.

Answer 2

According to Formula 26.3.19 of Abramowitz and Stegun's Handbook of Mathematical Functions,

$$\int_0^\infty\int_0^\infty f(x,y;\rho)\;\mathrm dx\;\mathrm dy = \frac 14 + \frac{\arcsin \rho}{2\pi}$$ where $f(x,y;\rho)$ is the bivariate normal density of two standard normal random variables with correlation coefficient $\rho$. By symmetry, $\frac 14 + \frac{\arcsin \rho}{2\pi}$ is also the value of the joint CDF at the origin. Since $\arcsin\rho$ increases monotonically from $-\frac{\pi}{2}$ to $+\frac{\pi}{2}$ as $\rho$ increases from $-1$ to $+1$, this proves the desired result.