Standardized GARCH-residuals, distributions and AIC

Question

So, I have been wondering about an interesting observation. My data contains 1006 log-returns of the SP500-index and I've estimated a GARCH(1,1)-process with Gaussian quasi-maximum likelihood - eventhough the logreturns is fitted best by a Student's t-distribution.

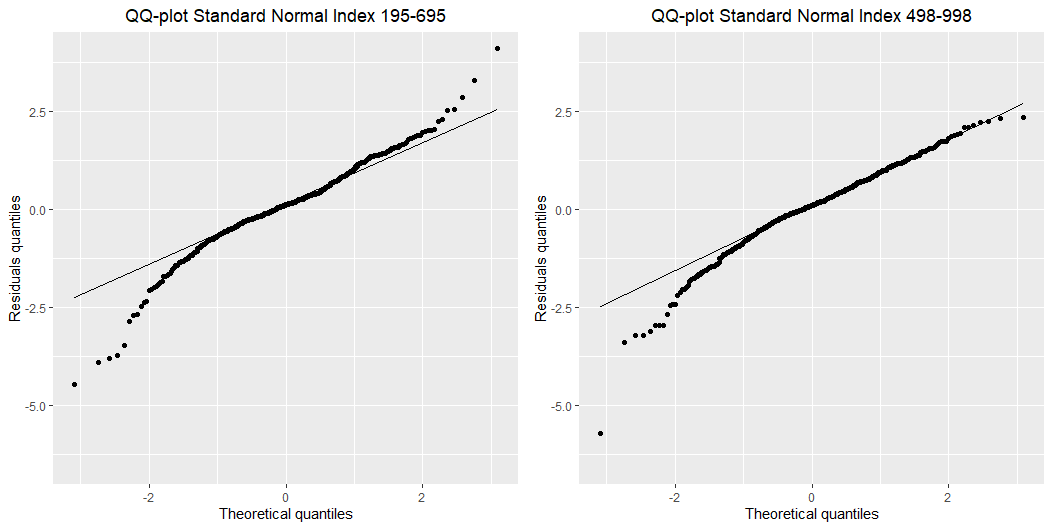
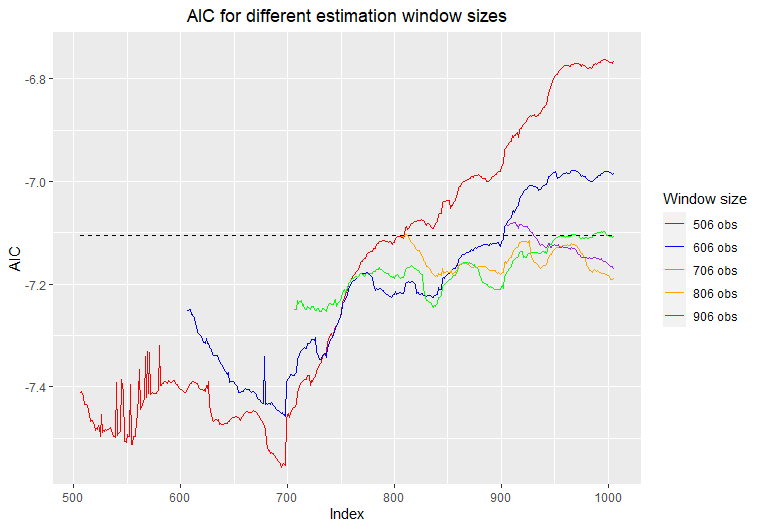
I was interested in some goodness of fit arguments and was wondering about different periods of my data and how the GARCH(1,1)-process fits the different periods. I found out the best AIC-value was produced by the indices 195-695 (500 observations fitted) $$\text{AIC}_{\text{best}}=-7.556248$$ and the worst produced by the indicies (498-998) $$\text{AIC}_{\text{worst}}=-6.763304.$$ But when I look at the densities of the standardized residuals from those two periods and QQ-plots against a standard normal distribution my result is quite disturbing and I cannot find the intuition behind it.

This is the log-returns plot I'm looking at: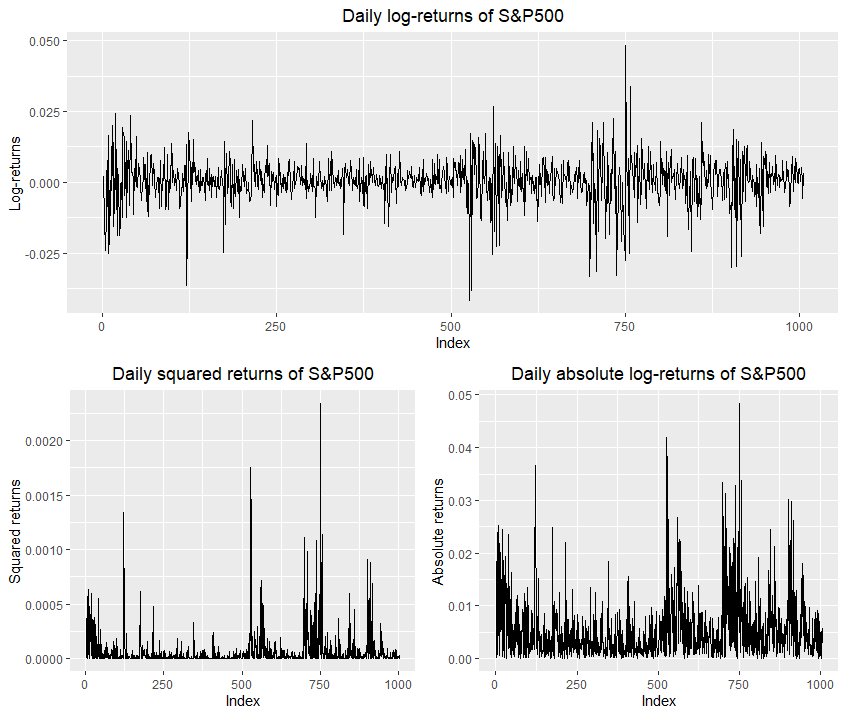

And these are the QQ-plots:

I was thinking that it might had something to do with the stationarity. It's clear that in the period of indicies 195-695 I have "more" stationarity in the period of indicies 468-998. But since I use Gaussian quasi-maximum likelihood (assuming the noise process is standard Gaussian), how do one explain the "bad" fit on the "good" standardized residuals? Thank you in advance.

AIC plot

Answer 1

AIC is a measure of likelihood (more precisely, $-2n\ \times$ expected log-likelihood of a the model for a new observation from the same population).

For a fixed dataset, the better the assumed distribution matches the actual distribution, the higher the likelihood. Thus, if you were only looking at a single window of your dataset and had two models based on different distributional assumptions, you would expect the model with the higher likelihood to also have the assumed distribution of residuals matching the empirical distribution better.

For different datasets, however, the likelihoods will be incomparable. Hence, you need not expect to see a positive relationship between (1) size of the likelihood and (2) how well the distributional assumptions are matched when looking at different windows of your dataset.

Below is an empirical example in R illustrating the point.

par(mfrow=c(1,2)) # plot two graphs in one

# True error distribution uniform, assumed distribution normal, high value of log-likelihood
n=1e2                       # set sample size
set.seed(1); x=runif(n)     # fix seed and generate regressor x
set.seed(0); u=runif(n)/10  # fix seed and generate true error term u
y=0+1*x+u                   # generate y from x and u
m=lm(y~x)                   # estimate a linear regresion y~x
e=m$resid                   # obain residuals
hat_sigma_e=sqrt(mean(e^2)) # MLE of sigma_e
loglik=sum(log(dnorm(e,mean=0,sd=hat_sigma_e))) # log-likelihood
# Alternatively, run logLik(m)
loglik                      # print log-likelihood
plot(y~x,main=paste("Wrong distributional assumption \n Log-likelihood =",round(loglik,2)))
points(m$fitted~x,col="red")
lines(m$fitted~x)

# True error distribution normal, assumed distribution normal, low value of log-likelihood
n=1e2 # set sample size
set.seed(1); x=runif(n)     # fix seed and generate regressor x
set.seed(0); u=rnorm(n)*10  # fix seed and generate true error term u
y=0+1*x+u                   # generate y from x and u
m=lm(y~x)                   # estimate a linear regresion y~x
e=m$resid                   # obain residuals
hat_sigma_e=sqrt(mean(e^2)) # MLE of sigma_e
loglik=sum(log(dnorm(e,mean=0,sd=hat_sigma_e))) # log-likelihood
# Alternatively, run logLik(m)
loglik                      # print log-likelihood
plot(y~x,main=paste("Correct distributional assumption \n Log-likelihood =",round(loglik,2)))
points(m$fitted~x,col="red")
lines(m$fitted~x)