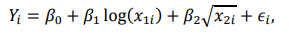I'm still not quite sure what differentiates the two, and what it means to be "linear within the parameters"
1
-
$\begingroup$ I like Jeff Miller's video on nonlinear basis functions. $\endgroup$– DaveCommented Aug 13, 2021 at 17:23
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
The model is linear in $(\log(x_{1i}), \sqrt{x_{2i}})$. It would normally be fitted using methods for linear models. So most people would probably call this linear, but the distinction between linear and nonlinear models is to some extent fuzzy, and you might find people who say this is nonlinear in $(x_{1i},x_{2i})$ that can be made linear by transformation - it's good practice to say linear/nonlinear in what, if you want to be precise.
answered Aug 13, 2021 at 16:37
-
1$\begingroup$ "Linear in the parameters" has a different meaning: it means the model is linear in $(\beta_0,\beta_1,\beta_2).$ $\endgroup$– whuber ♦Commented Aug 13, 2021 at 17:01