I'm reading a lecture slide that starts by asking if there's a way to invert a characteristic function $\psi_X$ if $\int|\psi_X(t)|~\mathrm{d}t = \infty$. From my reading, the slide then provides a proof sketch of the inversion formula. This proof sketch states to assume you can use a theorem (proposition 13 here).
Why ask about the integrability of $\psi_X$, but then assume just that?
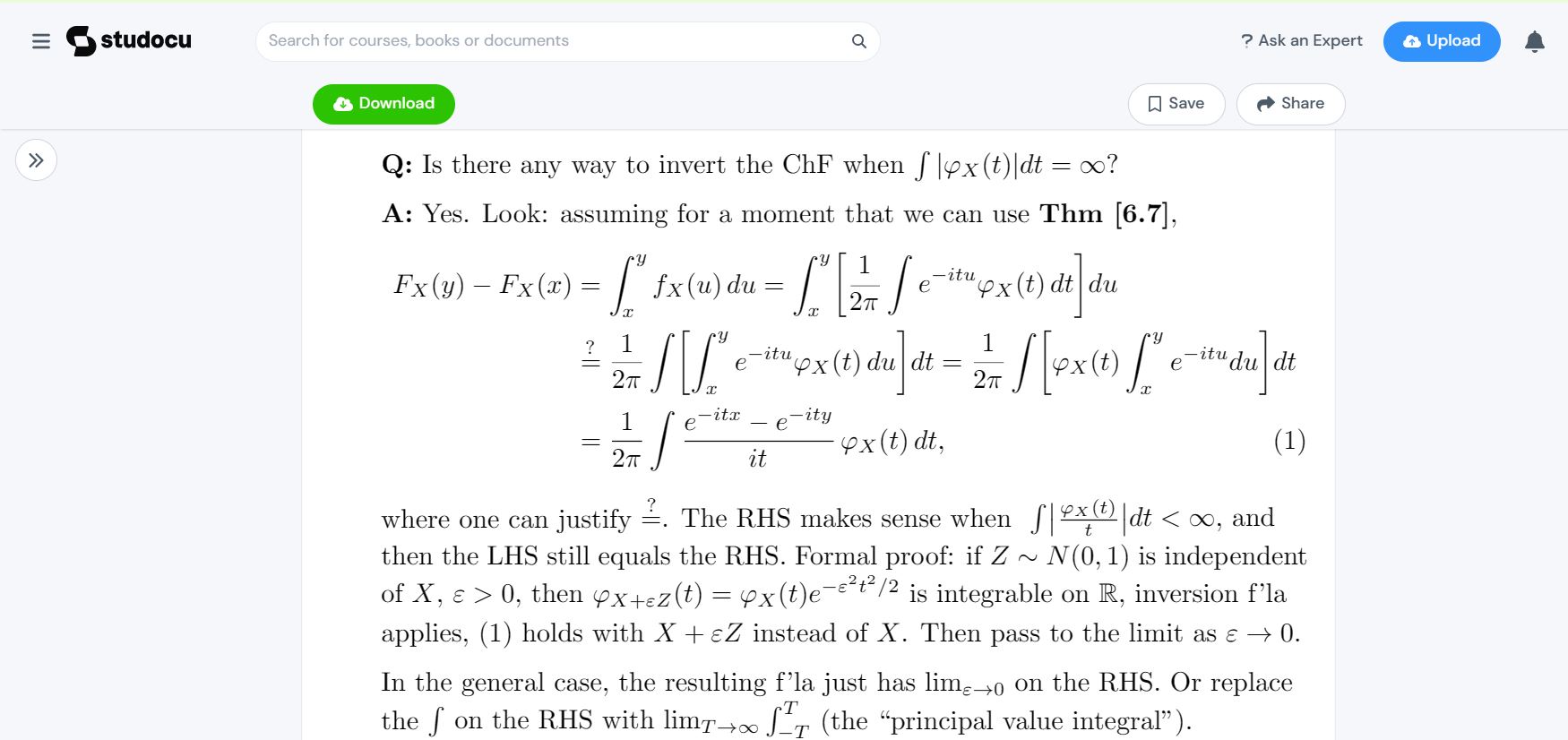
It doesn't make sense to use $f(x)=\frac{1}{2\pi}\int \exp(-\mathrm itx) \varphi_X(t)~\mathrm dt$ when $\int|\varphi_X(t) |~\mathrm dt=\infty, $ for the former is true if $\int|\varphi_X(t) |~\mathrm dt<\infty.$
The general inversion formula (assuming $a,~b\in \mathcal C(\mathrm F) ;~a<b$) is
$$ \mathrm F(b) - \mathrm F(a) =\lim_{T\to\infty}\frac1{2\pi}\int_{-T}^T\frac{\exp{(-\mathrm ita) }-\exp{(-\mathrm itb) }}{\mathrm it}\varphi_X(t)~\mathrm dt. \tag 1\label 1$$ The deduction of $\eqref 1$ involves showing the integral $$\mathscr I_T:=\frac1{2\pi}\int\left[\int_{-T}^T\frac{\exp{(\mathrm it(x-a) ) }-\exp{(\mathrm it(x-b) ) }}{\mathrm it}~\mathrm dt\right]~\mathrm d\mathrm F(x) $$ being bounded ( by $|b-a|$).
As is emphasized in $\rm [I], $ when $\int|\varphi_X(t) |~\mathrm dt<\infty,$ then only the integral in $\eqref 1$ can be extended over $\mathbb R. $ Subsequently we reach from $\eqref 1$ the more usual inversion formula.
$\rm [I]$ Probability and Measure, Patrick Billingsley, John Wiley and Sons, $1986, $ sec. $26, $ pp. $355-357.$
