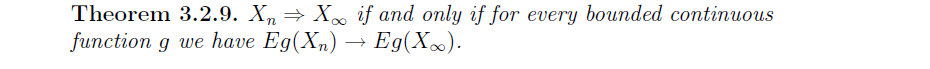3
$\begingroup$

The theorem to prove is that if $X_n$ converges weakly to $X$, and $P(X \in D_g) = 0$ where $D_g$ is the set of discontinuity of $g$, then $g(X_n)$ converges weakly to $g(X)$.

In Durrett, this is proved by using the a.s. representation, getting $Y_n$ that equals to $X_n$ in distribution and $Y_n \to Y$ almost surely.

enter image description here

In contrast, Wikipedia does not use a.s. representation

enter image description here

enter image description here

As far as I can tell both proof uses the same assumption, but the proof of Wikipedia skips using a fairy strong result of being able to find $Y_n$ such that $Y_n = X_n$ in distribution and $Y_n \to Y$ in distribution.

So is there something wrong with the proof in Wikipedia? If not, why did Durrett present a "harder" proof?

Theorem 3.2.9 just says that $X_n \to X$ weakly if and only if for all continuous and bounded $f$, $E(f(X_n)) \to E(f(X))$.

enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
8
$\begingroup$

The Wikipedia's proof is not fully rigorous and incomplete. It is not fully rigorous because as we allow $g$ can have discontinuities, the statement "$F = f \circ g$ is itself a bounded continuous functional" is an overstatement. It is incomplete because it failed to explicitly cite the bounded convergence theorem (as Durrett's book did) or any other propositions to close the argument "And so the claim follows from the statement above". Because it skipped this important step which relies on the Skorohod's theorem (i.e., the "a.s. representation" in your post) to prepare the convergence condition in BCT, it created the illusion that its "proof" is simpler.

The application of the Skorohod's theorem in Durrett 's proof to continuous mapping theorem is very elegant, and the same idea is also shared by Billingsley (see Theorem 25.7 in Probability and Measure). However, if you think such proof used too much machinery, you can directly verify other equivalence conditions of weak convergence (portmanteau lemma). For example, to check $$\limsup_{n \to \infty} P(g(X_n) \in F) \leq P(g(X) \in F)$$ for every closed set $F$. A proof of this kind can be found in Theorem 2.3 of Asymptotic Statistics by A. W. Van der Vaart.

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Billingsley explained in a much better and meaningful way the derivation than Durrett. His explanation of the quantile function, then the theorem followed by the mapping theorem, the overall presentation is more intuitive. $\endgroup$
    – User1865345
    Commented Mar 10, 2023 at 17:42
  • 1
    $\begingroup$ @User1865345 I guess what you meant is that in terms of the overall arrangement of a set of relevant theorems, Billingsley did a better job than Durett? If restricting the scope to the proof of the continuous mapping theorem itself, I think both authors presented it equally clear -- although Billingsley's proof is more direct and shorter (in that he actually didn't invoke the portmanteau lemma and BCT), Durett's BCT proof is also witty. $\endgroup$
    – Zhanxiong
    Commented Mar 10, 2023 at 18:25
  • $\begingroup$ Yes. In a overall setting too, Billingsley seems to be more slow paced in breaking down the theorems in relevant bits rather than condensing. This of course is my opinion. And yes, Durrett's summoning of BCT was indeed witty. But I prefer the treatment of Billingsley which have been followed by the other books that I follow. $\endgroup$
    – User1865345
    Commented Mar 10, 2023 at 18:29
  • 1
    $\begingroup$ Finally, I would rather take days to turn pages of various books rather than satisfy myself with Wikipedia. :-) $\endgroup$
    – User1865345
    Commented Mar 10, 2023 at 18:31
7
$\begingroup$

Zhanxiong has already elaborated on what Durrett is up to and what the Wikipedia article missed.

However let me emphasize the fact that the application of (Baby) Skorohod Theorem rather is ingenious and makes the deduction extremely easier than what would have been otherwise.

To give an outline:

$\bullet$ If $\mathrm F_n\Rightarrow \mathrm F, $ for $t\in (0, 1) \cap \mathcal C(\mathrm F^\leftarrow) ,$ then $\mathrm F_n^\leftarrow (t) \to \mathrm F^\leftarrow(t). $

$\bullet$ Using this, show there exists, if $X_n\Rightarrow X$ on a probability space, $X^\#_n,X^\#$ on $([0, 1], \mathcal B([0, 1]), \lambda) $ such that $X_n\overset{\mathrm d}{=}X_n^\#$ and $X_n^\#\overset{\mathrm{a.s.}}{\to}X^\#.$ This is possible by defining $X_n^\#:= \mathrm F_n^\leftarrow(U) $ where $U$ is uniformly distributed. (Baby Skorohod Thoerem)

$\bullet$ For any map $h:\mathbb R\mapsto \mathbb R$ such that $\mathbb P[X\in \mathrm{Disc}(h) ]=0,$ it is easy to check $h\left(X_n^\#\right) \overset{\textrm{a.s.}}{\to} h\left(X^\#\right) $ w.r.t. $\lambda.$ As almost sure convergence implies weak convergence, then $$ h(X_n) \overset{\mathrm d}{=}h\left(X_n^\#\right)\Rightarrow h\left(X^\#\right)\overset{\mathrm d}{=} h(X).$$

As $\rm [II]$ sums up:

Once again, this is a pretty and easy proof. BUT it relies on a very sophisticated prerequisite. In other words, we do not get anything for free.

References:

$\rm [I]$ A Probability Path, Sidney Resnick, Birkhäuser, $1999, $ sec. $8.3, $ pp. $259-261.$

$\rm [II]$ Probability: A Graduate Course, Allan Gut, Springer Science$+$Business, $2005, $ sec. $5.13.1, $ pp. $258-260.$

Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ The last quote perfectly pointed out what Wikipedia missed and answered the question. +1. $\endgroup$
    – Zhanxiong
    Commented Mar 10, 2023 at 18:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.