23
$\begingroup$

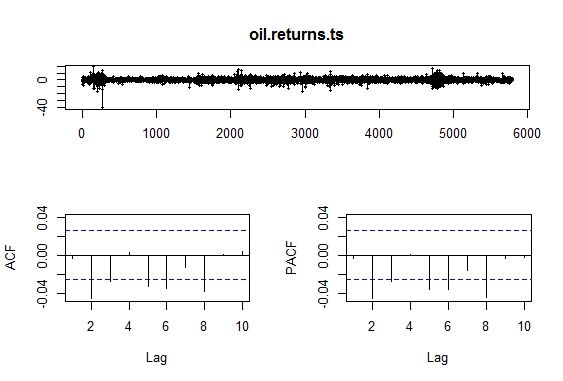
Returns

So I plotted the ACF/PACF of oil returns and was expecting to see some positive autocorrelation but to my surprise I only get negative significant autocorrelation. How should I interpret the above graph? They seem to indicate that there is a tendency for oil returns to increase when it decreased previously and vice-versa, thus the oscillating behaviour. Please correct me if I'm wrong.

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
10
$\begingroup$

Negative ACF means that a positive oil return for one observation increases the probability of having a negative oil return for another observation (depending on the lag) and vice-versa. Or you can say (for a stationary time series) if one observation is above the average the other one (depending on the lag) is below average and vice-versa. Have a look at "Interpreting a negative autocorrelation".

Improve this answer
$\endgroup$
4
  • 3
    $\begingroup$ In practice, however, the autocorrelations you show are all very small: although some are significant at conventional levels they should not be over-interpreted. Correlations about 0.02 don't imply much predictive ability. $\endgroup$
    – Nick Cox
    Commented Oct 26, 2013 at 15:37
  • $\begingroup$ Would it make sense if I tried fitting an ARMA-GARCH model to this dataset?Would it make sense to use ARMA for correlation this small?I know I can just fit the return in a GARCH model but I don't want to end up with constant 0 when forecasting return. $\endgroup$
    – ankc
    Commented Oct 26, 2013 at 17:32
  • $\begingroup$ @Stat, can you answer the above questions please? thanks $\endgroup$
    – ankc
    Commented Oct 28, 2013 at 7:37
  • $\begingroup$ Sorry Andy, I thought I have answered them. Well, you can try both of them, and then check your models to see which one fits better to the returns and provides a reasonable forecast. But as Nick said, you don't have that much correlation, and that makes it difficult to find a good time series model. $\endgroup$
    – Stat
    Commented Oct 28, 2013 at 15:20

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.