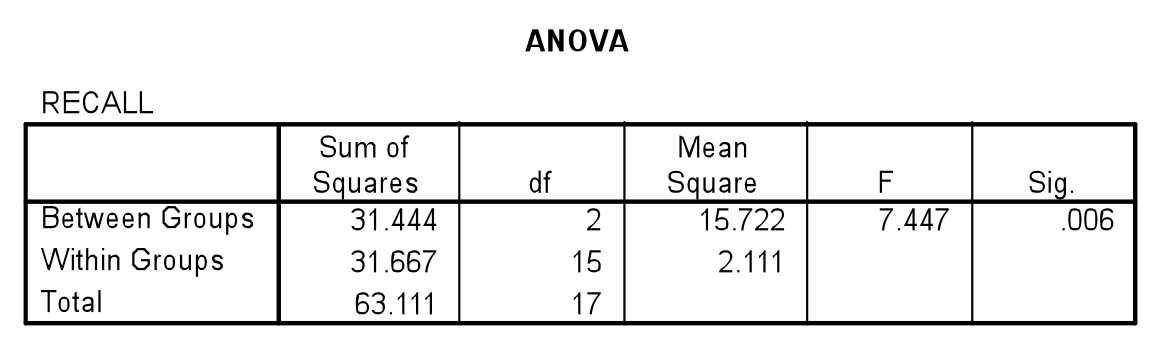
I realize that the divisions are meant to take into account the number of factor levels (which will tend to push up $\text{SS}_\text{between}$), and the number of observations (which will tend to push up $\text{SS}_\text{within}$). However, I can't understand why we don't obtain these Mean Squares by dividing $\text{SS}_\text{between}$ by k and $\text{SS}_\text{within}$ by N.
For example, in the following data there were three levels to the factor, and 18 observations in total.
Under the null hypothesis (and the assumptions of ANOVA), $SS_\text{between}$ has expected value $(k-1)\, \sigma^2$ and $SS_\text{within}$ has expected value $(N-k)\, \sigma^2$.
(the reason why the sum of $N$ residuals-squared has expectation $(N-k)\, \sigma^2$ rather than $N \sigma^2$ is because each group's sample mean is closer to the data (in sum of squares sense) than the true mean is.
[If the alternative hypothesis is the case, the numerator has a larger expected value, but the denominator's expected value is unchanged. This is why we reject when $F$ is large.]
So when you divide by the appropriate degrees of freedom, both MS terms are estimates of the same quantity, $\sigma^2$, the variance of the error term when the null is true.
Taking their ratio then cancels out the $\sigma^2$, leaving something typically close to 1 under the null hypothesis (and typically larger than 1 under the alternative) and which has an F-distribution.
