I have made the following model in DAGitty:

Where X2 is controlled for.

DAGitty says:

The total effect cannot be estimated due to adjustment for an intermediate or a descendant of an intermediate.

I asked here whether it would be possible to obtain the treatment effect after controlling for x2.

But I guess my fundamental question is: if I control for both x2 and x1, why, from the theory of DAGs, that doesn't identify the treatment effect?

I mean, controlling for x2, which is a collider, open a backdoor path x1-y. But controlling for x1 should close this backdoor path again.

Why, from a theoretical perspective, that doesn't happen?

I will reinforce my understanding of how that should work with another example.

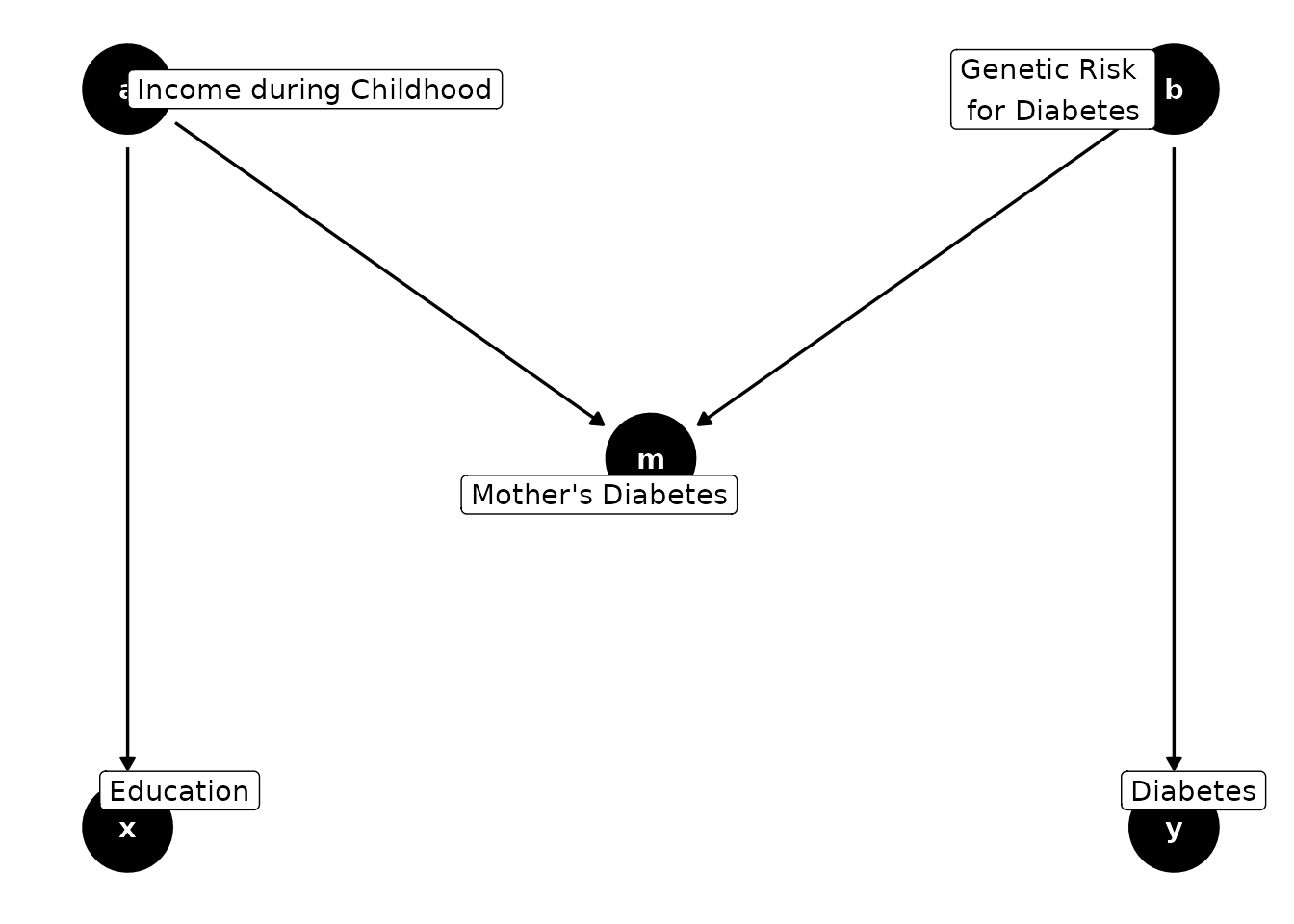
Consider the following DAG:

x is the treatment, and y is the outcome.

In this DAG I can control for nothing.

Or I can control for {m,a}, or for {m,b}, or for {m,a,b}.

In fact, m is a collider, and controlling for it induces a backdoor path x-a-m-b-y.

But I can close the backdoor path so opened by controlling for also a, or for also b, or for also a and b together.

Why that doesn't happen with the first DAG I posted?

If I control for x2 (the collider), and so open the backdoor path t-x1-x2-y, why I can't close the backdoor path so opened by controlling for x1?

I would like an answer from a theoretical point of view.