Does sphericity (an RM-ANOVA assumption) require the covariances between difference scores to be the same?

Question

Field's Discovering Statistics Using SPSS (2013, Sage) defines sphericity as follows:

Sphericity: a less restrictive form of compound symmetry which assumes that the variances of the differences between data taken from the same participant (or other entity being tested) are equal. This assumption is most commonly found in repeated-measures ANOVA but applies only where there are more than two points of data from the same participant.

This definition doesn't mention anything about the covariances between difference scores needing to be equal. Is that because the definition is incomplete, or because the covariances don't need to be equal? Why is it necessary/not necessary for covariances to be equal?

I found here a comment stating that sphericity implies the difference variables should have the same covariances as each other:

Sphericity implies that "difference variables" (i.e. with 3 RM levels these are: RM1-RM2, RM1-RM3, RM2-RM3) have the identity covariance matrix

I'm thinking here about a repeated-subjects ANOVA.

Here is a table from Field (2013) that illustrates what I mean by difference scores.

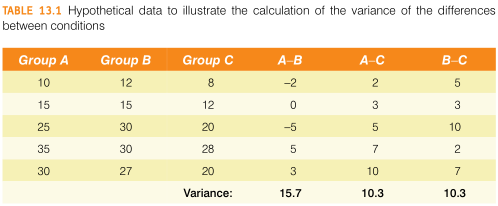
As noticed in the comments, there is an error in the book. I've assumed that the first value in Group C is really 8, and that therefore B-C should be 4, and Variance B-C should be 10.7.

I have calculated the covariances between the difference scores (not the actual scores) and they are as follows:

A-B, A-C 7.65  
A-B, B-C -8.05  
A-C, B-C 2.65

It turns out that the variances of the difference scores are a bit dissimilar (15.7, 10.3, 10.7). However, let's imagine those variances were exactly the same as each other (15.7, 15.7, 15.7), but the three covariances remained very different from one another. Would sphericity thereby be violated? Or are the covariances between difference scores not relevant to sphericity? Why are they relevant or not relevant to sphericity?

Answer 1

No, it does not.

The assumption of sphericity in RM-ANOVA is usually formulated as in your quote from Field:

the variances of the differences between [RM factor levels] are equal

You can also check e.g. An Introduction to Sphericity, or any reference therein.

Perhaps one can think that this requirement somehow implies that all the covariances between the differences are going to be equal too. This is not the case, as I will demonstrate via an example.

Here is a $4\times4$ covariance matrix between the factor levels, satisfying the sphericity assumption, as presented in the above link:

$$\begin{pmatrix}10& 5& 10& 15\\ 5& 20& 15& 20\\ 10& 15& 30& 25\\ 15& 20& 25& 40\end{pmatrix}$$ It clearly does not satisfy compound symmetry, but it does satisfy sphericity. Indeed, we can easily compute the $6\times 6$ covariance matrix between all pairwise differences using $$\newcommand{\Cov}{\operatorname{Cov}}\Cov(A-B,C-D)=\Cov(A,C)-\Cov(B,C)-\Cov(A,D)+\Cov(B,D).$$

Here is my Matlab code to do it:

S = [10 5 10 15; 5 20 15 20; 10 15 30 25; 15 20 25 40];
diffs = [1 2; 1 3; 1 4; 2 3; 2 4; 3 4];

for i=1:size(diffs,1)
    for j=1:size(diffs,1)
        SD(i,j) = S(diffs(i,1),diffs(j,1)) + S(diffs(i,2),diffs(j,2)) - ...
            S(diffs(i,1),diffs(j,2)) - S(diffs(i,2),diffs(j,1));
    end
end

Here is the result:

$$\begin{pmatrix} 20 & 10 & 10 & -10 & -10 & 0\\ 10 & 20& 10& 10& 0& -10\\ 10 & 10 & 20 & 0 & 10 & 10\\ -10 & 10 & 0 & 20 & 10 & -10\\ -10 & 0 & 10 & 10 & 20 & 10\\ 0 &-10 &10 &-10 &10 &20\end{pmatrix}$$

All the values on the diagonal are equal to $20$, hence the sphericity assumption is satisfied. But off-diagonal values are $10$, $-10$, and $0$, i.e. they are not the same.

---

A reader might wonder why the assumption of equal variances of all differences is called "sphericity", given that none of the covariance matrices presented above is actually spherical (see my answer in What is an isotropic (spherical) covariance matrix?).

This is to be discussed in a separate follow-up thread: Why is the "sphericity assumption" in RM-ANOVA (constant variance of difference scores) called "sphericity"?

Answer 2

As to covariances, the variance of a difference is $\sigma^2_{x-y}=\sigma^2_x+\sigma^2_y-2\sigma_{xy}$, so covariances are somewhat involved, but they don't need to be equal.
Sphericity requires that $$\sigma^2_{i-j}=\sigma^2_i+\sigma^2_j-2\sigma_{ij}=k$$ (the same $k$) for all $i,j$. This doesn't require equal covariances. See this page for an example. This question and my answer could be useful too.

EDIT: In your example there are $N=5$ observation and $J=3$ groups, so checking for sphericity is simple, you just have to compute 15 differences (and 3 variances). If the number of observations were larger, say $N=100$, you should compute 300 differences. Using variances and covariances you just need $J=3$ variances and $J(J-1)/2=3$ covariances. In R:

> A <- c(10,15,25,35,30)
> B <- c(12,15,30,30,27)
> C <- c(8,12,20,28,20)
> # variances
> var(A)
[1] 107.5
> var(B)
[1] 74.7
> var(C)
[1] 60.8
> # covariances
> cov(A,B)
[1] 83.25
> cov(A,C)
[1] 79
> cov(B,C)
[1] 62.4

So the variance-covariance matrix is: $$\begin{bmatrix} 107.5 & 83.25 & 79 \\ 83.25 & 74.7 & 62.4 \\ 79 & 62.4 & 60.8 \end{bmatrix}$$ Now you can check for sphericity: $$\begin{split} Var(A-B) &= 107.5+74.7-2\cdot83.25=15.7 \\ Var(A-C) &= 107.5+60.8-2\cdot 79=10.3 \\ Var(B-C) &= 74.7+60.8-2\cdot 62.4=10.7 \end{split}$$

In the first method you don't need covariances. You need them in the second method. The above algebra shows that:

• if the variances are equal, then the covariances must be equal too (this is compound symmetry, which imply sphericity);
• if the variances are not equal, then the covariances must vary so that the above sums are equal.