How does a Poisson distribution work when modeling continuous data and does it result in information loss?

Question

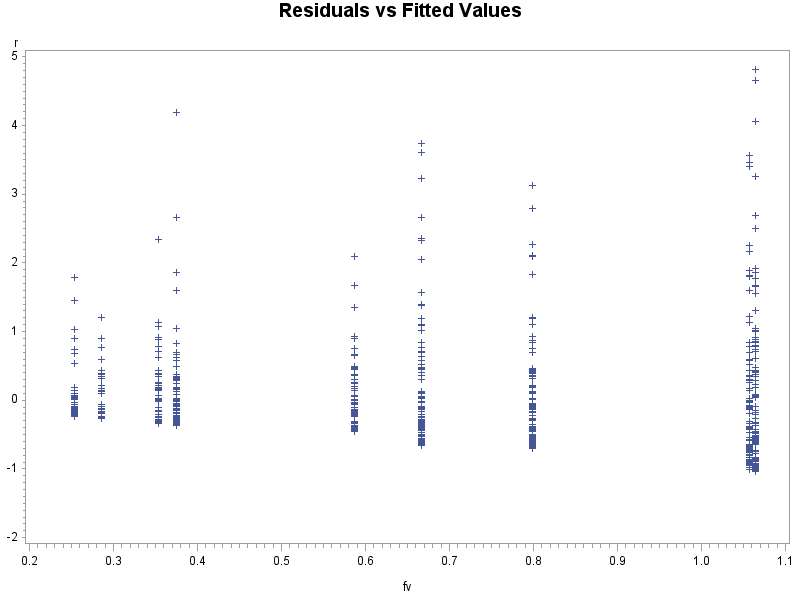
A co-worker is analyzing some biological data for her dissertation with some nasty Heteroscedasticity (figure below). She's analyzing it with a mixed model but is still having trouble with the residuals.

Log-transforming the response variables cleans things up and based on feedback to this question this seems to be an appropriate approach. Originally, however, we had thought there were issues in using transformed variables with mixed models. It turns out that we had been misinterpreting a statement in Littell & Milliken's (2006) SAS for Mixed Models that was was pointing out why it is inappropriate to transform count data and then analyze it with a normal linear mixed model (full quote is below).

An approach that also improved the residuals was to use a generalized linear model with a Poisson distribution. I've read that the Poisson distribution can be used for modeling continuous data (eg, as discussed in this post), and stats packages allow it, but I don't understand what is going when the model is fit.

For the purpose of understanding how the underlying calculations are being made, my questions are: When you fit a Poisson distribution to continuous data, 1) does it the data get rounded to the nearest integer 2) does this result in the loss of information and 3) When, if ever, is it appropriate to use a Poisson model for continuous data?

Littel & Milliken 2006, pg 529 "transforming the [count] data may be counterproductive. For example, a transformation can distort the distribution of the random model effects or the linearity of the model. More importantly, transforming the data still leaves open the possibility of negative predicted counts. Consequently, inference from a mixed model using transformed data is highly suspect."

Answer 1

I've been estimating continuous positive outcome Poisson regressions with the Huber/White/Sandwich linearized estimator of variance fairly frequently. However, that's not a particularly good reason to do anything, so here are some actual references.

From the theory side, $y$ does not need to be an integer for for the estimator based on the Poisson likelihood function to be consistent. This is shown in Gourieroux, Monfort and Trognon (1984). This is called Poisson PMLE or QMLE, for Pseudo/Quasi Maximum Likelihood.

There's also some encouraging simulation evidence from Santos Silva and Tenreyro (2006), where the Poisson comes in best-in-show. It also does well in a simulation with lots of zeros in the outcome. You can also easily do your own simulation to convince yourself that this works in your snowflake case.

Finally, you can also use a GLM with a log link function and Poisson family. This yields identical results and placates the count-data-only knee jerk reactions.

References Without Ungated Links:

Gourieroux, C., A. Monfort and A. Trognon (1984). “Pseudo Maximum Likelihood Methods: Applications to Poisson Models,” Econometrica, 52, 701-720.

Answer 2

Poisson distribution is for count data only, trying to feed it with continuous data is nasty and I believe should not be done. One of the reasons is that you don't know how to scale your continuous variable. And the Poisson depends very much on the scale! I tried to explain it with a simple example here. So For this reason alone I'd not use Poisson for anything other than count data.

Also remember that GLM does 2 things - link function (transforming the response var., log in Poisson case), and residuals (Poisson distrubution in this case). Think about the biological task, about the residuals, and then select proper method. Sometimes it makes sense to use log transform, but stay with normally distributed residuals.

"but it seems like conventional wisdom is that you shouldn't transform data entering into a mixed model"

I hear this first time! Doesn't make any sense to me at all. Mixed model can be just like a normal linear model, just with added random effects. Can you put an exact citation here? In my opinion, if log transform clears things up, just use it!

Answer 3

Here's another great discussion of how to use the Poisson model to fit the log-regressions: http://blog.stata.com/2011/08/22/use-poisson-rather-than-regress-tell-a-friend/ (I am telling a friend, just as the blog entry suggests). The basic thrust is that we only use the part of the Poisson model that is the log link. The part that requires the variance to be equal to the mean can be overridden with a sandwich estimate of the variance. This is all for i.i.d. data, however; the clustered/mixed-model extensions have been properly referenced by Dimitriy Masterov.

Answer 4

If the problem is the variance scaling with the mean, but you have continuous data, have you thought about using continuous distributions that can accomodate the issues you're having. Perhaps a Gamma? The variance will have a quadratic relationship with the mean - much like a negative binomial, actually.