As explained on this Wikipedia page, if two random variables X and Y are uncorrelated and jointly normally distributed, then they are statistically independent.
I know how to check whether X and Y are correlated, but have no idea how to check whether they are jointly normally distributed. I hardly know any statistics (I learnt what a normal distribution is a couple of weeks ago), so some explanatory answers (and possibly some links to tutorials) would really help.
So my question is this, having: Having two signals sampled a finite number of N times, how can I check whether the two signal samples are jointly normally distributed?
For example: the images below show the estimated joint distribution of two signals, s1 and s2, where:
x=0.2:0.2:34;
s1 = x*sawtooth(x); %Sawtooth
s2 = randn(size(x,2)); %Gaussian
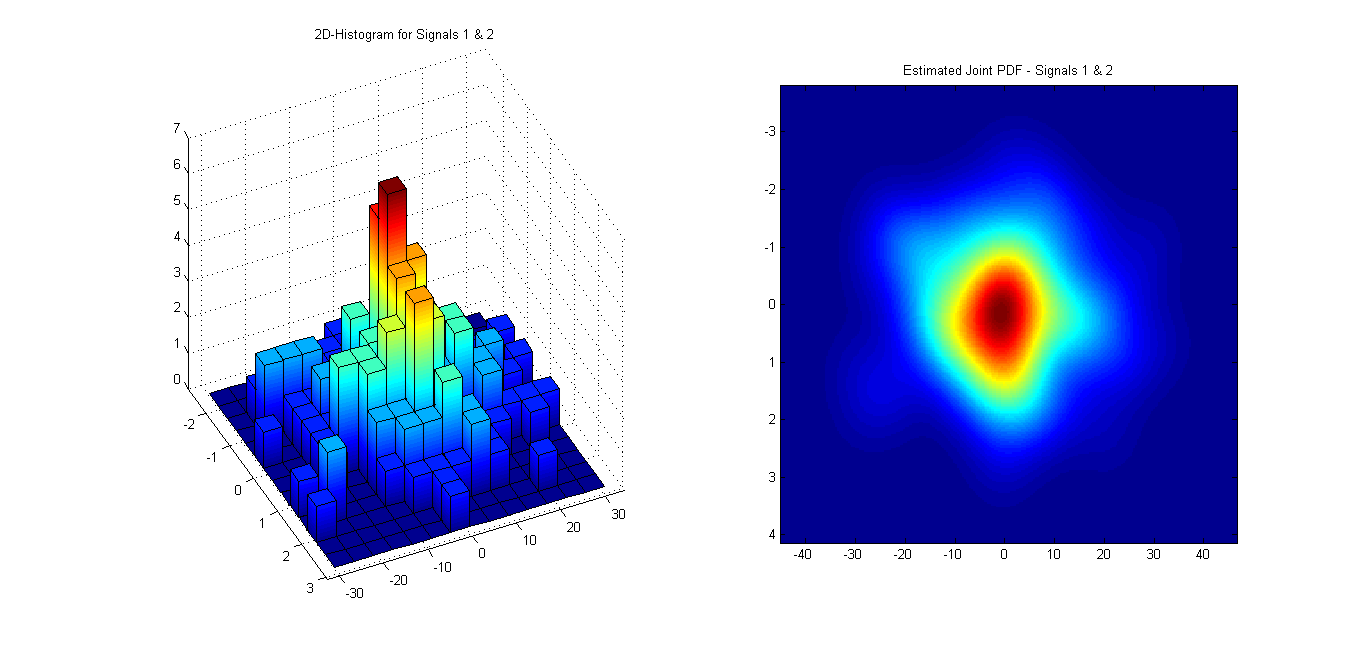
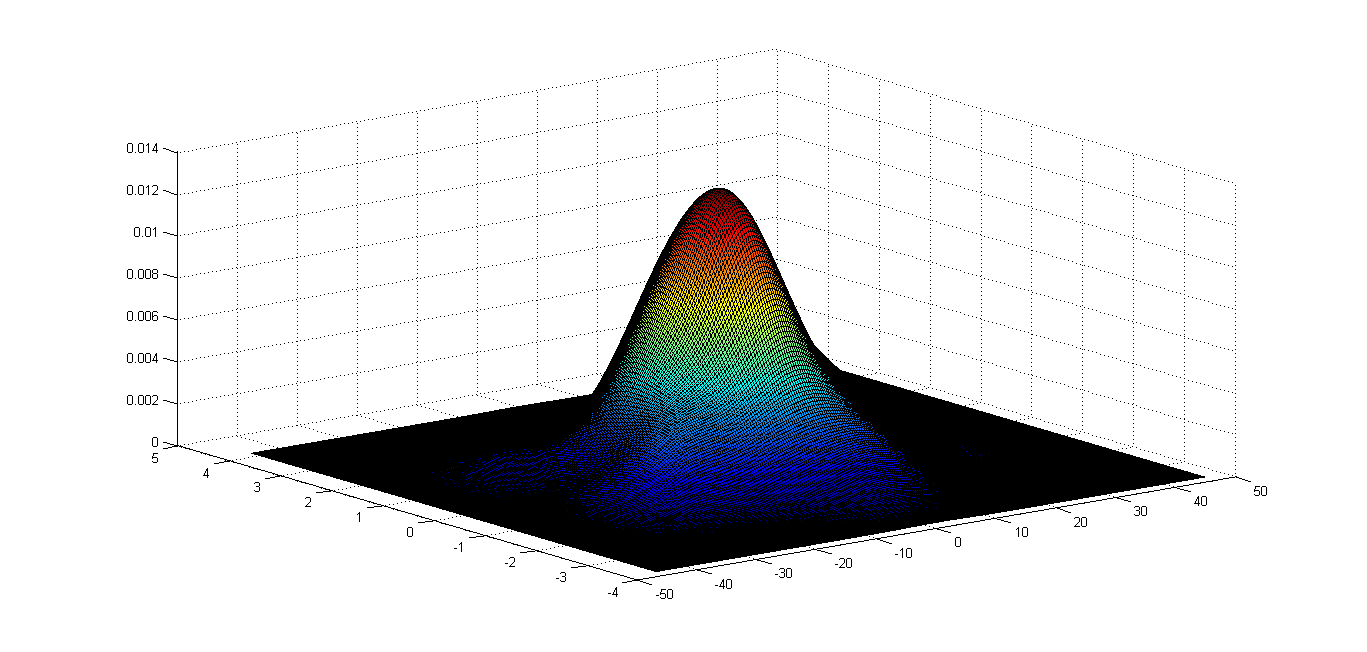
The joint pdf was estimated using this 2D Kernel Density Estimator.
From the images, it is easy to see that the joint pdf has a hill-like shape centred approximately at the origin. I believe that this is indicative that they are in fact jointly normally distributed. However, I would like a way to check mathematically. Is there some kind of formula that can be used?
Thank you.
