I have a dataset composed of 2 factors of several levels each (i.e. exercise[A-B-C] x muscle [1-2-3-4-5-6]. I'm interested on the effect of the different exercises (A,B,C) on the muscle activation amplitude (i.e. 6 different muscles).
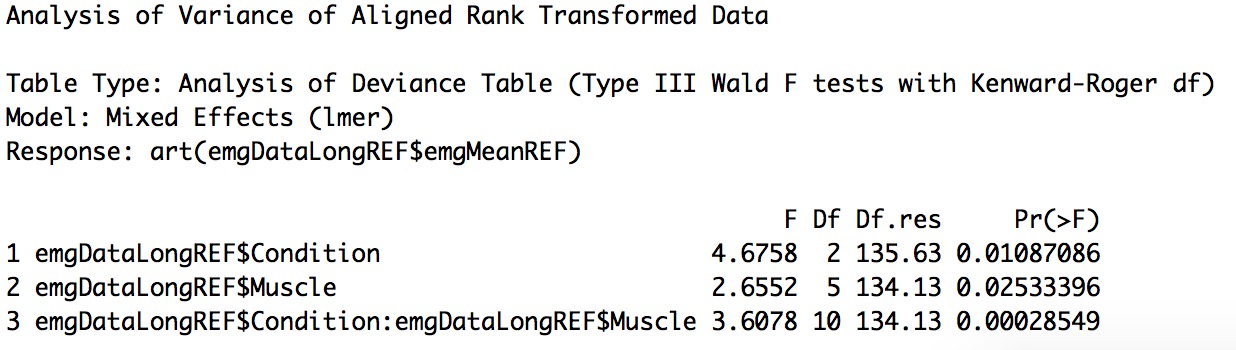
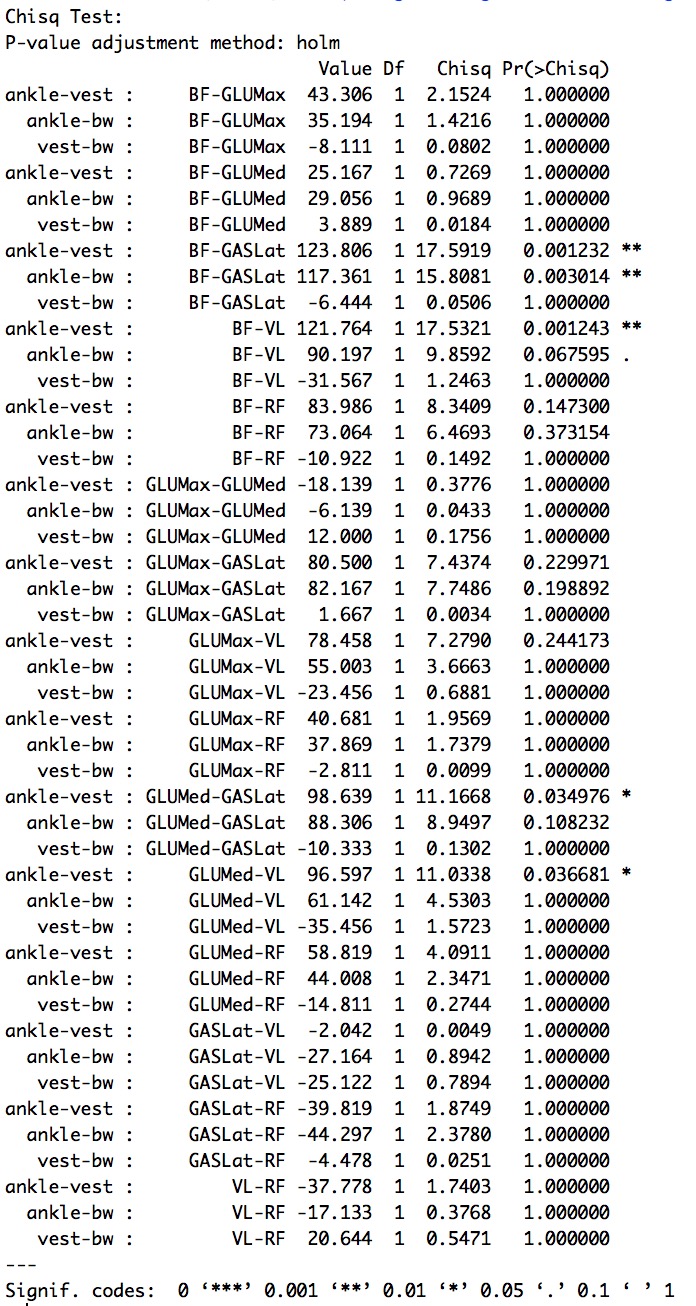
After performing the Analysis of Variance of Aligned Rank Transformed Data I found a significant interaction exercise x muscle. Thus, I tested (post hoc) differences of differences for the interaction term (pairwise comparisons).
I obtained some significant results but I'm struggling with the interpreation. Below an example of the statistical outcome:
A - B : 1 - 3 p = 0.001 ***; A - C : 1 - 3 p = 0.003 **; B - C : 1 - 3 p = 1;
Basically, we can ask, is the difference A - B different when muscle = 1 compared to when muscle = 2? So, that seems ok but what does it mean practically?